SHORT QUESTIONS- X (All chapter)
GOODS AND SERVICES TAX
Multiple Choice Questions
1) IGST is charged on:
a) interstate transaction b) intrastate transaction c) both a, b d) none
2) GST payable is equals to
a) ITC --output GST b) output GST + ITC c) output GST - ITC d) output GST
3) A dealer in Mumbai sold a washing machine to a consumer in Mumbai for Rs18000. If the rate of GST is 18% and GST, then SGST is:
a) Rs1620 b) Rs3240 c) nil d) none
4) In a transaction from Delhi to Lucknow, MRP = Rs10000, discount= 10%, GST= 28%. Here IGST is:
a) Rs2520 b) Rs5040 c) nil d) none
5) A refrigerator was sold for Rs15000 under intrastate transaction from station A to station B and the GST rate is 18%. CGST is:
a) Rs1450 b) Rs 1350 c) Rs1300 d) Rs2700
6) A dealer purchased goods worth Rs 15000 and sold them for Rs21000 within the state. If the rate of GST is 12%, then the net SGST paid by the dealer is:
a) Rs360 b) Rs720 c) Rs800 d) Rs850
7) A dealer in Delhi sold a water heater whose marked is Rs22000 to a customer in Delhi at discount of 25%. If the rate of GST 18%, then the SGST paid is:
a) Rs 1485 b) Rs2970 c) Rs1980 d) nil
8) Anushka bought 400g of almonds at Rs1200 per kg. If the rate of GST is 5%, the amount paid by Anushka is:
a) Rs504 b) Rs480 c) Rs1260 d) Rs630
9) A dealer in Agra sold an LED to a customer in Agra for Rs28000. If SGST is Rs2420, then the rate of GST is:
a) 12% b) 18% c) 9% d)28%
10) Mr. Sharma purchased goods worth Rs40000 from a dealer(within the same state ). If the rate of GST 18%, then CGST is:
a) Rs 3600 b) Rs7200 c) Rs8000 d) nil
11) The tax invoice of a mobile company in Delhi shows cost of services provided by it as Rs1200. If the rate of GST is 18%, then amount of the bill is:
a) Rs 1400 b) Rs1308 c) Rs 1416 d) Rs1500
12) A shopkeeper in Rampur sold an oven to a customer in Rampur for Rs26400. If the rate of GST is 28%, then IGST is:
a) Rs3696 b) Rs7392 c) Rs1848 d) nil
13) A dealer in Bhopal (MP) supplies goods worth Rs 30000 to a dealer in Delhi. The dealer in Delhi supplies the same goods to a customer in Delhi at a profit of Rs5000. If the rate of GST is 18%, then the net GST paid by the dealer in Delhi is:
a) Rs900 b) Rs2700 c) Rs5400 d) nil
14) A dealer in Patna (Bihar) supplies goods worth Rs 15000 to a dealer in Sonepat(Haryana). The dealer in Sonepat supplies the same goods to a dealer in Rohtak (Haryana) at a profit of Rs 3000. If the rate of GST is 18%, then the net GST paid by the dealer in Sonepat is:
a)Rs 540 b) Rs700 c) Rs720 d) nil
15) A retailer purchases an iron for Rs1500 from a dealer and sells it to a consumer at 10% profit. If the sales and intrastate , and the rate of GST is 12%, then the selling price of the iron including GST by the retailer is:
a)!Rs1550 b) Rs1848 c) Rs1950 d) Rs
16) A shopkeeper purchased a fan for Rs1500 from a dealer and sold it to a customer at 10% profit. If the sells are intrastate and the rate of GST is 12%, then the tax (under GST) received by the central government is :
a) Rs18 b) Rs99 c) Rs198 d) nil
17) Goods from Delhi are sold to Ranchi(Jharkhand) for Rs20000 and then from Ranchi to Cuttack (Odisha). If the rate of GST is 18%, and the profit made at Ranchi is Rs5000, then the net GST payable by the dealer in Ranchi is :
a) Rs1000 b) Rs900 c) Rs850 d) Rs875
18) A shopkeeper bought an article from a dealer at Rs1000. He sold it to the customer at Rs1200. If the rate of GST is 12%, then the amount paid by the customer to buy the item is:
a) Rs 1200 b) Rs1300 c) Rs1344 d) Rs1350
19) Three friends A, B and C live in Delhi. A sales medicine worth Rs50000 to B, B sells the same medicine to C at a profit of Rs 6000. If the rate of GST is 12%, then SGST paid by B is:
a) Rs300 b) Rs360 c) Rs400 d) Rs425
20) Mr Gupta wanted to book a semidelux room in a hotel for Rs750. Since semidelux room was not available, he booked a delux room for Rs1400. If GST for a room below Rs1000 is 18% and GST for a room above Rs1000 is 28%, then the extra GST paid by Mr Gupta for the delux room is:
a) Rs 257 b) Rs280 c) Rs300 d) Rs425
Answer:
1a 2c 3a 4a 5b 6a 7a 8a 9b 10a 11c 12 d 13 a 14a 15b 16b 17b 18c 19b 20a
Short Answer Type Questions:
1) Find the amount of bill for the following intrastate transaction of goods , if the GST rate is 28%.
items I II III
Marked price(Rs) 7000 14700 28200
discount% 20 10 10
2) A registered garment house in Ludhiana (Punjab) sold three lots of garments to a dealer in Bhatinda (Punjab) for Rs1000000; Rs600000 and Rs500000. It also charged Rs10000 on each lot of transportation charges . But on the occasion of Diwali , a discount of 1% was given on each lot . If the rate of GST is 5%, Calculate
a) IGST
b) CGST
c) SGST
3) Saurav went to watch a new movie in a multiplex. He wanted to buy a movie ticket for Rs80, but it was not available. So, he bought a ticket for Rs120. If the GST for a ticket below Rs100 is 18%, and GST for a ticket above Rs100 is 28%, how much extra GST and extra amount did saurav pay for the ticket?
4) A dealer in Rohtak (Haryana) bought a gold ring from a manufacturer in Gurgaon (Haryana) for Rs800000. He sold this ring to a dealer to Nainital (Uttarakhand) for Rs95000. If the rate of GST is 3% find
a) the new GST payable at Rohtak
b) Input tax credit (ITC) for dealer in Nainital.
5) A retailer in Jaipur( Rajasthan) buys goods from a dealer in Alwar (Rajasthan) at a discount 20%. The retailer sales it to a customer in Jaipur at the printed price. If the printed price of the goods is Rs16000 and the GST rate is 8%, calculate :
a) the prince paid by the customer for the goods.
b) the CGST and SGST payable by the retailer in Jaipur to the government.
Answer
1) Rs56588.80 2) 0, Rs52725, Rs52725 3) Rs19.20, Rs59.20 4) Rs450, Rs2850 5) Rs17280, 128, 129
LONG ANSWER TYPE QUESTIONS
1) An e-learning company in Delhi sets the marked price of an e-book as Rs75000. It sells the e-book to a dealer in Patna (Bihar) at discount of 30%. The dealer in Patna raises the marked price of the e-book by 30% and then sells it to a dealer in Ranchi (Jharkhand). if the rate of GST is 5%, find the GST paid by the dealer in Patna to the government.
2) A manufacturer in Noida (UP) sold a cartoon of hair oil to a dealer in Rajpur (Chhattisgarh) for Rs22000. The dealer in Raipur sold it to a consumer in Bastar (Chhattisgarh) at a profit of Rs5000. If the rate of GST is 18%, find:
a) the net IGST , CGST and SGST payable by the dealer in Raipur.
b) the cost price of the hair oil for the customer.
3) A dealer in Jodhpur (Rajasthan) supplied floor tiles worth Rs1700000 to a dealer in Delhi and another worth Rs300000 to a dealer in Jaipur (Rajasthan). The total value of his receipt of tiles in interstate transactions was Rs1500000. If the rate of GST is 18%, calculate the net IGST , CGST and SGST payable by the dealer in Jodhpur.
4) A shopkeeper sells an item for Rs2150. For a customer, he reduced the price of the item in such a way that the customer has to pay only Rs2124 including GST. If the rate of GST is 18%. Calculate the amount of reduction allowed by the shopkeeper .
5) A fruit juice company in Allahabad (UP) sold fruit juice worth Rs16000 to a dealer in Hapur(UP). The whole lot of juice was then supplied to a dealer in Rudrapur (Uttarakhand) for Rs17500. If the rate of GST is 12%. Find :
a) the net GST payable at Hapur.
b) input Tax credit (ITC) for the dealer in Rudrapur.
6) Radheshyam is a dealer of footwear in Moradabad (UP). He purchase footwear worth Rs200000. He sold 50% of thess footwear to a dealer in Agra (UP) for Rs130000 and the rest of the stock remains in his godown. If the rate of GST is 5%, find the excess credit of CGST and SGST to be carried forward.
7) The marked price of a video camera Recording is Rs80000. A dealer in Delhi buys it from a dealer in Mumbai (Maharashtra) at discount of 20% on the marked price . The dealer then sells it to a customer in Rohtak (Haryana) at a discount of 10% on the marked price. if the rate of GST is 18%, calculate the amount of tax payable by the dealer in Delhi to the government.
Answer
1) Rs2250 2) Rs0, Rs0, Rs900, Rs31860 3) Rs36000, Rs27000, Rs27000 4) Rs350 5) Rs180, Rs2100 6) Rs750, Rs750 7) Rs1440
BANKING
1) Sujata has a recurring deposit account in a bank for 2 years at 6% p.a, simple interest. If she gets Rs1200 as interest at the time of maturity, then her monthly installment is :
a) Rs500 b) Rs600 c) Rs700 d) Rs800
2) A man deposited Rs1000 per month in a recurring deposit account for 3 years at 8% p.a, The maturity value is:
a) Rs440000 b) Rs 40000 c) Rs40440 d) Rs44444
3) Abhinav deposited Rs200 per month for 3 years in a bank's recurring deposit account. If the bank pays intrest at the rate of 11% p.a., then the interest at the time of maturity is:
a) Rs1210 b) Rs1221 c) Rs1415 d) Rs1521
4) Kamal has a recurring deposit account in a bank for 2 years at 6% p.a., S. I. if he gets Rs1350 as interest, then the monthly installment is:
a) Rs1000 b) Rs900 c) Rs850 d) Rs
5) if Kavita deposited Rs900 per month for 2 years and 6 months in a recurring deposit account, then the total money she deposited in the account is:
a) Rs 26000 b) Rs27000 c) Rs28000 d) Rs30000
6) A man deposited RsX per month for Y years in a recurring deposit account. If at the time a maturity he got RsZ as interest, then the total maturity amount is:
a) Rs(12XY + Z) b) Rs 12 X Y Z c) Rs( X Y + 12Z) d) Rs XYZ/12
7) Karan deposited Rs2000 per month for 3 years in a recurring deposit account . if the rate of interest is 11% p.a., the amount he gets on maturity is:
a) Rs72000 b) Rs80000 c) Rs82210 d) Rs 84210
8) A man deposited Rs2000 per month in a recurring deposit account for 18 months at 8% p.a, The interest he will get at the time of maturity is :
a) Rs2280 b) Rs2350 c) Rs2400 d) Rs2410
9) Mr Awasthi has a 4 year time deposit account and deposits Rs 650 per month. If he received Rs5096, as interest at the time of maturity, the rate of interest is:
a) 8% p.a b) 8.5%p.a c) 9%p.a d) 10%p.a
10) Sharda deposited Rs150 per month in a bank for 8 months under the recurring deposit scheme. If the rate of interest is 8% p.a., then intrest earned at the time of maturity is:
a) Rs50 b) Rs40 c) Rs36 d) Rs30
11) Ravi has a 4 year cumulative time deposit account and deposits Rs650 per month. If he receives Rs36296 at the time of maturity , the rate of interest was :
a) 10% p.a b) 9%p.a c) 8%p.a d) 7.5%p.a
12) Piyush has a recurring deposit account for 2 years at 10%p.a. If he receives Rs1900 as intrest , the monthly installment paid by him is:
a) Rs700 b) Rs750 c) Rs760 d) Rs800
13) A man deposited Rs1500 every month in a bank for 8 months under the recurring deposit scheme. If the rate of interest is 8%p.a, than the interest earned at the time of maturity is:
a)Rs 400 b) Rs350 c) Rs36⁰ d) Rs320
14) Kamal deposits Rs4000 per month in a recurring deposit account for 3/2 years at 6%p.a. The intrest he will receive at the time of maturity is:
a)Rs 3420 b) Rs3240 c) Rs3150 d) Rs3110
15) Garima deposited Rs500 per month in a recurring deposit account for 3 years. If the rate of interest is 4%p.a, then the amount she gets at the time in maturity is:
a) Rs1110 b) Rs19110 c) Rs 20150 d) Rs21110
16) Amrita deposited Rs1600 per months in a recurring deposit account for 2 years. If the rate of interest is 8% p.a, then the interest earned by her at the time of maturity is:
a) Rs 3200 b) Rs3450 c) Rs3550 d) Rs3600
17) Manisha deposited Rs500 per month recurring deposit account for 2 years. If the bank pays interest at 8%p.a, then the interest she gets at the time of maturity is:
a) Rs 1000 b) Rs1100 c) Rs 1200 d) Rs1500
18) Ajay deposited Rs2400 per month for 18 months in a bank's recurring deposit account. If the bank pays interest at 6%p.a, the interest he gates at the time of maturity is:
a) Rs 1550 b) Rs18000 c) Rs2052 d) Rs3250
19) Sameer has a recurring deposit account in the bank for 3 years at 4% simple interest. If he gets Rs4440 as interest at the time of maturity, then the monthly installment is:
a) Rs2000 b) Rs26000 c) Rs3000 d) Rs3200
20) Dinesh has a recurring deposit account, which pays interest at 5%p.a. if he pays Rs 2500 per month for 2 years, then the interest he will get at the time and maturity is
a) Rs 3000 b) Rs3025 c) 3200 d) Rs3125
1d 2c 3b 4b 5b 6a 7d 8a 9a 10c 11c 12c 13c 14a 15b 16a 17a 18c 19a 20d
Short Answer Type Questions:
1) Rekha opened a recurring deposit account for 20 months. The rate of interest is 9% per annum and Rekha receives Rs441 as interest at the time of maturity. Find the amount Rekha deposited each month.
2) Mr Sonu has a recurring deposit account and deposited Rs750 per month for 2 years. If he gets Rs19125 at the time of maturity, find the rate of interest.
3) Kabeer opened a recurring deposit account in a bank and deposited Rs300 per month for 2 years. If he received Rs7725 at the time of maturity, find the rate of interest per annum.
4) Ram deposits Rs1000 per month in a recurring deposit account for 3 years at 8% per annum intrest. Find the maturity value.
5) Reema deposited Rs200 per month for 3 years in a bank' recurring deposit account. If the bank pays interest at the rate of 11% p.a., find the amount she gets on maturity.
6) Harishankar opened a recurring deposit account in a bank and deposited Rs800 per month for 18 month. If he received Rs15084 at the time of maturity, find the rate of interest per annum.
Answers
1) Rs280 2) 6% 3) 7% 4) Rs40440 5) Rs8421 6) 6%
Long Answer Type Questions
1) Mohan has a recurring account in a bank for 2 years at 6% simple interest. If he gets Rs1200 as intrest at the time of maturity, find
a) the monthly installment.
b) the amount of maturity.
2) Priyanka has a recurring deposit account of Rs1000 per month at 10% per month. If she gets Rs5550 as interest at the time of maturity, find the total for which the account was held.
3) Manish opened a recurring deposit account in a bank. He deposited per month for two years. At the time of maturity, he got Rs67500. Find
a) the total interest earned by Manish.
b) the rate of interest per annum.
4) Mr Gupta has a recurring deposit account in a bank. He deposits Rs2500 per month for 2 years. If he gets Rs66250 at the time of maturity, find
a) the interest paid by the bank.
b) the rate of interest.
5) Mr Garg deposits a certain sum of money each month in a recurring deposit account of a bank. If the rate of interest is 8% p.a and Mrs Garg gets Rs 8088 from the bank after 36 months, find the value of his monthly installment.
5) Vandana has recurring time deposit account of Rs340 per month at 6% p.a. If she gets Rs7157 at the time of maturity, find the total time for which the account was held.
Answers:
1) Rs8000, Rs20400 2) 3 years 3) Rs7500, 12% 4) Rs6250, 10% 5) Rs200 6) 20 months
SHARES AND DIVIDEND
1) The total amount of money needed to run the company is called:
a) shares b) capital c) dividend d) principle
2) The whole capital to run a company is divided into small units, called:
a) Shares b) Share holders c) face value d) dividend
3) The annual profit distributed among share holders is called:
a) nominal value b) market value c) dividend d) face value
4) The value of a share printed on the share certificate is called its :
a) nominal value b) market value c) discount d) below par
5) The shares of different companies can the brought or sold in the market through stock exchange. The price at which the share is share or purchased is called its
a) face value b) market value c) par value d) Nominal value
6) A share is said to be____, if its market value is the same as its face value.
a) premium b) discount c) par c) nominal value
7) A share is said to be at premium, if its market value is ____then its face value.
a) more b) less c) same d) equal
8) A share is said to be____, if its market value is less than its face value.
a) At par b) above par c) below par d) premium
9) The face value of a share
a) change every year b) changes from time to time c) always remains the same d) none to
10) Dividend is always paid on the____ of a share.
a) market value b) face value c) investment d) dividend
11) The market value of a share.
a) never changes b) changes from time to time c) changes every month d) none
12) Number of share hold by a person=
a) total nominal value/face value of one share
b) total market value/ face value of one share.
c) dividend/market value of one share.
d) dividend/investment x 100
13) dividend =
a) number of shares x nominal value
b) number of shares x market value
c) face value x number of shares x rate of dividend/100
d) none
14) Rate of return on investment=
a) investment/dividend
b) dividend/investment
c) dividend/ investment x 100
d) investment/dividend x 100
15) investment/ sale proceeds =
a) number of shares x market value
b) number of shares x nominal value
c) face value x number of shares x rate of dividend
d) dividend/investment
16) annual income of a share holder
a) number of shares x face value
b) number of shares x rate of dividend x face value of 1 share
c) number of shares x market value x face value
d) market value x nominal value x 100
17) If a share of of Rs100 is selling at Rs125, then it is said to be selling at a ___ of Rs25.
a) discount b) premium c) at par d) below par
18) if a share of Rs125 is selling at Rs96, then it is said to be selling at Rs 29___.
a) below par b) at par c) above par d) premium
19) if Kabir invests Rs 10320 on Rs100 shares at a discount of Rs14, then the number of shares he buys is
a) 110 b) 121 c) 150 d) 100
20) Shahrukh has some shares of Rs50 of a company paying 15% dividend. If his annual income is Rs 3000, then the number of shares he processes is
a) 400 b) 600 c) 800 d) 200
21) If Kiran invests Rs19250 on Rs50 shares at a premium of 20%, then the number of shares she buys is
a) 640 b) 160 c) 320 d) 240
22) Varun possesses 600 shares of Rs25 of a company. If the company announces a dividend of 8%, then his annual income is
a) Rs600 b) Rs1200 c) Rs480 d) Rs120
23) A man invests Rs24000 on Rs60 shares at a discount of 20%. If the dividend declared by the company is 10%, then his annual income is
a) Rs2880 b) Rs1500 c) Rs3000 d) none
24) Rs25 shares of a company are selling at Rs20. If the company is playing a dividend of 12%, then the rate of return is
a) 10% b) 18% c) 15% d) 12%
25) Rs 40 shares of a company are selling at 25% premium. If Mr Washim wants to buy 280 shares of the company, then the investment required by him is
a) Rs14000 b) Rs16800 c) Rs8400 d) Rs10000
1b 2a 3c 4a 5b 6c 7a 8c 9c 10b 11b 12a 13c 14c 15a 16b 17b 18a 19b 20a 21c 22b 23c 24c 25a
SHORT ANSWERS:
1) Find the % return on investment in
a) 6% Rs100 shares at Rs120
b) 25/3% Rs100 share at Rs150
2) Find the price of 5% Rs100 shares when an investor gets a dividend of Rs65 by investing Rs1430.
3) At what price should a 25/4% Rs 100 shares be quoted when the money is worth 5%
4) A person invested Rs1284 in 8/2% Rs100 shares . His dividend was Rs54. Calculate the M.V of the shares.
5) A company pays 7% dividend . For how much should a man buy a Rs100 shares for getting 8% on his investment.
6) Which share is more profitable: 4% Rs100 share at Rs80 or 9/2% Rs100 share at Rs88 ?
7) A part of Rs3020 is invested in 6% Rs100 shares at Rs97 and the rest in 12% Rs100 share at Rs108. If both bring the same dividend, find the sum invested in the share selling
a) at discount
b) above par
c) the total dividend
8) A person bought 360 ten rupee share paying 12% dividend at par and sold them at Rs21. The proceeds were invested in 5 rupees shares paying 9/2% at 3.5 per share. Find
a) sale proceeds
b) the number of 5 rupees shares bought
c) the percentage in income
9) A person invests Rs4368 in 100 rupees share at Rs91. Shares worth Rs24000 face value are sold at Rs95 and the rest at Rs85. Find
a) the number of shares bought Rs91
b) the number of shares sold at Rs85
c) the loss or gain in the deal .
10) Rs8000 and Rs 10000 were invested in Rs100 shares giving dividends 12% and 8% respectively. The dividend are collected and all the shares are sold at a loss of 2% and 3% respectively on the investment. Find
a) the dividend collected
b) the total sell proceeds
c) gain percent on the whole transaction.
1) 5%, 50/9% 2)Rs110 3) Rs125 4) Rs107 5) Rs87.50 6) 9/2 shares at Rs88 7) Rs1940, Rs1080, Rs240 8) Rs 7560, Rs 2160, 12.5% 9) 48,24, Rs 48 loss 10) Rs 1760, Rs 17540, 65/9%
LONG ANSWER
1) A man has a choice to invest in Rs 100 shares of two firms at Rs120 each or at Rs132 each. The first firm pays a dividend at 5% per annum and the second firms pays a dividend of 6% per annum. How much more will his annual return be if he invests Rs 26400 with the firm from which he gets a better return on his investment ?
2) A man bought one thousand shares each of face value Rs5 at Rs7 per share . At the end of the year, the company from which he bought the shares declared a dividend of 8%. Calculate
a) the amount of money invested by the man
b) the percentage return on his outlay. (Correct to one decimal place).
3) Ajay owns 560 share of a company. The face value of each share is Rs25. The company declares a dividend of 9%. Calculate
a) the dividend that Ajay will get.
b) the rate of interest on his is investment, if Ajay had to pay Rs30 for each share.
4) A company with 4000 shares of nominal value of Rs110 each declares an annual dividend of 15%. Calculate
a) the total amount of dividend paid by the company.
b) the annual income of shah Rukh who holds 88 shares of the company.
c) If he received only 10% on his investment, find the price Shah Rukh paid for each share.
5) Amit Kumar invests Rs36000 in buying Rs100 shares at Rs20 premium. The dividend is 15 percent per annum. Find
a) the number of shares he buys .
b) his yearly dividend
c) the percentage return on his investment
6) Vivek invested Rs45000 in 8% Rs10 shares at Rs15 sells the shares when the price rise to Rs30 and invests the proceeds in 12% Rs100 shares at Rs125. Calculate
a) the sale proceeds
b) the number of Rs125 shares he buys
c) the change in his annual income from dividend.
1) Rs 100 2) Rs 7000, 5.7% 3) Rs 1260, 7.5% 4) Rs 66000, Rs 1452, Rs 165 5) 300, 4500,12.5% 6) Rs 9000,72, Rs 624
LINEAR INEQUATIONS
1) If 2x - 7 < 4, where x is a natural number less than 8, than the solution set is:
a) {0,1,2,3,4} b) {1,2,3,4,5} c) {1,2,3,4,5,6} d) {0,1, 2,3,4,5,6}
2) If - x ≥ -3 then:
a) x≤ -3 b) x ≥ 3 c) x = 3 d) x ≤ 3
3) if 2 + 4 x< 2 x - 5 ≤ 3x ∈Z, then the solution set is :
a) {5,4} b) {- 5,-4} c) {- 5, -4, -3} d) {- 4,-3,-2,-1}
4) if 2≤2x - 3 ≤ 5, x∈ R, then the solution set is:
a) {2.5≤ x ≤ 4, x∈ R} b) {2≤ x ≤ 5, x∈ R} c) {3≤ x ≤ 5, x∈ R} d) {2< x < 4, x∈ R}
5) If a> b, then:
a) a - c ≤ b - c b) a - c≥ b - c c) a - c = b - c d) a - c > b - c
6) If x≥ 5 and- ax ≥ 5a, then :
a) a > 0 b) a < 0 c) both a and b d) neither a nor b
7) If x+1≥ 13 - 5x, x ∈{1,2,3,4.....10}, then the solution set is:
a) {1,2,3,4,5,6} b) {6,7,8,9,10} c) {7,8,9,10} d) {6,7,8.....}
8) If 7 - 5x ≥ 3x -1, then the solution set, when x ∈ W is:
a) {0,1} b) {0} c) {1} d) {0,1,2}
9) Given a >0, b >0, c >0 and d <0, then a < b implies :
a) a+ d> b + d b) a - d < b - d c) a - d > b - d d) a + d = b + d
10) Given 2x - 5≤ 5x +4 < 11. If x ∈ I, the solution set is:
a) {-2,-1,0,1} b) {-3,-2,-1,0,1} c) {-3,-2,-1,0} d) {-2,-1,0,1}
11) For the inequation -12< 3 - 4x ≤ 11, x ∈ N, the solution set on the number line can be shown as:
a) 5 b) 4 c) 3 d) 2
13) If 2x - 5≤ 5x + 4 < 11, x ∈ I, then the solution set can be represented as:
a) -2 b) -1 c) 0 d) 1
15) If -9(x -7)≥ 45 - 21x > x +1, x ∈ R, then the solution set is:
a) {-3/2≤ x < 2, x ∈R}
b) {-3/2 < x < 2, x ∈R}
c) {-2/3 ≤ x ≤ 1, x ∈R}
d) {-1/3 ≤ x ≤ 2, x ∈R}
16) If 2x - 5 ≤ 5x + 4 < 11, x ∈ I, then the smallest whole number for x is:
a) 0 b) 1 c) -3 d) 2
17) If 5 - 3x < 11, x ∈ R, then the solution set is:
a) {x> -2, x∈R} b) {x≥ -2, x∈R} c) {x< 2, x∈R} d) {x< -2, x∈R}
18) Given 3x -1 ≤ x +5, x ∈N, then the solution set is:
a) {1,2,3} b) {1,2,3,4} c) {1,2} d) {0,1,2,3}
19) If 8 < 5(x +1) -2 ≤ 18, x ∈R, then the smallest integer value of x is:
a) 1 b) 0 c) -1 d) 2
20) Given a >0, b >0, c >0 and d <0. Then a > b implies:
a) ad >bd b) ad = bd c) ad < bd d) none
1b 2d 3b 4a 5b 6a 7b 8d 9b 10b 11b 12b 13a 14a 15a 16a 17a 18a 19b 20c
SHORT ANSWER TYPE QUESTIONS
1) Find the value of x, which satisfies the inquation -2≤ 1/2 - 2x/3 ≤ 11/6, x ∈N.
Graph the solution set on the number line.
2) Solve the following inequation, write the solution set and represent it on the number line.
-3(x -7)≥ 15 - 7x > (x +1)/3, x ∈R
3) Solve the following inequation, write down the solution set and represent it on the real number line:
-2+ 10x ≤ 13x +10 < 24+ 10x, x ∈ Z
4) Solve the following inequation and write down the solution set:
11x - 4 < 15x +4 ≤ 13x + 14, x ∈ W
Represent the solution on a real number line.
5) Solve the given inequation and graph the solution set on the number line:
2y - 3 < y +1 ≤ 4y +7, y ∈ R
6) Solve the following inequation and represent the solution set on the number line:
2x -5 ≤ 5x +4 < 11, x ∈I
7) Solve the following inequation and write the solution set:
13x -5 < 15x +4 < 7x +12, x∈R
Answers:
1) x∈ {1,2,3} 2) {-1.5 ,≤ x < 2, x∈R} 3) {-4,-3,-2,-1,0,1,2,3,4} 4) {0,1,2,3,4,5} 5) {-2≤ y< 4, y∈ R} 6) {-3,-2,-1,0,1}
LONG ANSWER TYPE
1) Solve: - 31/3 < 5y/3 + 3 ≤ y/2 + 16/3, y ∈R
Graph the solution set on the number line.
2) Solve the inequation and represent the solution set on the number line:
-3 + x ≤ 8x/3 + 2 ≤ 14/3 + 2x, x∈ I
3) Solve the following inequation and represent the solution set on the number line:
- 3 < 1/2 - 2x/3 ≤ 5/6 , x ∈ R
4) Solve the following inequation and represent the solution set on the number line:
4x - 19< 3x/5 - 2 ≤ -2/5 , x∈ R
5) Solve the inequation, write the solution set and represent it on the number line:
-x/3 ≤ x/2 - 4/3 < 1/6, x ∈ R
6) Find the value of x which satisfy the inequation:
-17/6 < 1/2 - 2x/3 ≤ 2, x ∈W.
Graph the solution set on the number line:
7) Solve the following inequation, write the solution set and represent it on the number line:
2x -1 ≥ x + (7 -x)/3 > 2, x ∈ R.
Answers:
1) {-8< y< 2, y∈ R}
2) {-3,-2,-1,0,1,2,3,4}
3) {-2 ≤ x < 15/4, x∈R}
4) {-4 ≤ x < 5, x∈ R}
5) {1.6 ≤ x < 3, x∈ R}
6) {0,1,2,3,4}
7) {x ≥ 5/2, x∈ R}
QUADRATIC EQUATION
1) If x²= 3x, then
a) x= 0 b) x=0 or x=3 c) x=3 d) x=0 and x= 3
2) If 3x²+ 8= 10x, then
a) x= 2 or 4/3 b) x=2 or x=3 c) x=3 or 4/3 d) x=1 and x= 1/3
3) The quadratic equation whose solution set is {-2, 3} is
a) x²- 3x -6=0 b) x²- x + 6=0 c) x² +x -6=0 d) x²- x -6=0
4) One of the roots of 2x²- 7x +6=0, is
a) -2 b)2 c)!-3/2 d) 4
5) The discriminant of the quadratic equation 2x²- x +3 =0 is:
a) 24 b) 25 c) -23 d) -20
6) On solving x² +4x - 21=0, we get
a) x= -3, or -7 b) x=7 or -3 c) x =7 or 3 d) x =-7 or 3
7) The roots of the quadratic equation 2x² + x x - 1 =0 are
a) 1/2 or -1 b) -1/2 or 1 c) -1/2 or -1 d) 1/2 or 1
8) The discriminant of x²- 4x - 7 =0 is:
a) 44 b) √44 c) 12 d) -12
9) The quadratic equation whose roots are -1, -5 is:
a) x² +6x +5 =0 b) x²- 6x +5 =0 c) x²+ 6x - 5=0 d) x²- 6x - 5=0
10) For the equation 3x²- 4x - 2 =0, the roots are:
a) real and equal b) real and unequal c) imaginary d) both (a) and (b)
11) For the quadratic equation 2x²- 4x + 1 =0, the discriminant is:
a) 0 b) +ve c) -ve d) none
12) For the quadratic equation 2x²- 3x + 1 =0, the discriminant is:
a) 1 b) -1 c) 0 d) 9
13) For the quadratic equation 9x²+ 6x +1 =0, the discriminant is :
a) +ve b) -ve c) 0 d) imaginary
14) For the quadratic equation 2x² + ax - a²=0, the sum of the roots is:
a) a/2 b) -a/2 c) 2a d) -a
15) Given a quadratic equation mx²+ 8x -2 =0, m≠ 0. For this quadratic equation the value of discriminant is:
a) 64- 8m b) √(64+ 8m) c) 64+ 8m d) √(8m - 64)
16) For the quadratic equation 3x²+ 7x + 8 =0, the roots are:
a) real and distinct b) real and equal c) imaginary d) both (a) and (b)
17) The roots of the quadratic equation 2x²- kx + k =0 are equal . If k ∈N, then k is
a) 0 b) 8 c) 6 d) -6
18) Given the quadratic equation x² + 2√2x +1 =0. The roots of the quadratic equation are:
a) (-√2±1)/2 b) (√2±1)/2 c) (√2±1) d) (-√2±1)
19) The quadric equations (m +1)x² + 2(m +3)x +(m +8) =0. has equal roots. The value of m is :
a) 1/3 b) 1/4 c) 3 d) -3
20) The quadratic equation x² + m(2x + m -1)+ 2 =0 has equal roots . The value of m is
a) 1 b) 2 c) -2 d) 0
Answers:
1b 2a 3d 4b 5c 6d 7a 8a 9a 10b 11b 12a 13c 14b 15c 16c 17b 18d 19a 20b
SHORT ANSWER TYPE QUESTIONS
1) Find the value of k for which the following equation has equal roots. x² + 4kx + (k²- k+2) =0.
2) Find the value of p for which the equation px² - 5x +p =0. has real and equal roots.
3) For what values of m the equation 2x² + mx +2 =0 has real roots ?
4) Find the values of p for which the equation px² + 2x +1 =0 has distinct real roots.
5) Show that the equation 2(p²+ q²)x² + 2(p+ q)x +1 =0 has no real roots when p≠ q.
6) Solve the equation x² - 4x - 8=0 for x. Give your answer correct to 3 significant figures.
7) Solve 3x² + 11x +10 =0, when x belongs to I by factorization.
8) Solve : √(2x +9)= 13 - x by factorization.
9) Solve the equation 2x² + √5 x -5 =0 using formula, write your answer correct two decimal places.
10) Solve for x and give your answer correct to 2 decimal places.
(x +1)/(2x +5) = (x +3)/(3x +4).
Answers:
1) -1 or 2/3 2) ±5/2 3) m≥ 4 or m ≤ -4 4) p<1 5) 5.46, -1.46 7) -2 8) 8 9) 1.12, -2.33 10) 5.87, -1.87
Long Answer Type Questions
1) For what values of k will be the following quadratic equation (k +1)x² - 4kx +9 =0 have real and equal roots. Solve the equation.
2) Solve: √(2x² -2x + 21) =2x -3.
3) Solve: 2{x/(x +1)}² - {5/(x +1)}+ 2 = 0, x≠ 1.
4) If a. b c are rational, prove that the roots of the equation (b - c)x² + (c - a)x +(a - b) =0 are also rational.
5) The difference of the squares of two natural number is 84. The square of the larger number is 25 times the smaller number. Find the numbers .
6) The sum of the ages of Vivek and his younger brother Amit is 47 years . The product of their ages in years is 550. Find their ages.
7) An aeroplane travelled a distance of 400 km at an average speed of x km/hr. On the return journey the speed was increased by 40 kmph. Write down an expression for the time for:
a) the onward journey.
b) the return journey
If the return journey took 30 minutes less than the onward journey write an equation in X and find the value of x.
8) The hotel bill for a number of people for overnight stay is Rs14400. If there were 4 more people, the bill each person had to pay would have reduced by Rs600. Find the number people staying overnight .
9) A fruitseller bought x apples for Rs1200.
a) Write the cost price of each apple in terms of x.
b) If 10 apples were rotten and he sold rest at Rs3 more than the cost price of each, write the selling price of (x -10) apples.
c) If he made a profit of Rs60 in this transaction , form an equation in x and solve it to evaluate x.
Answers:
3 or -3/4, 3/2,3/2 or ±6
2) 6 3) -2,1 5) 10,4 6) 22 years, 25 years 7) 400/x, 400/(x +40), x²+ 440x - 32000=0, 160
8) 8
9) 1200/x, (x - 10)(1200/x +3), x²- 3x - 4000=0, 80
RATIO AND PROPORTION
1) If x², 4 and 9 are in continued proportion, then the value of x is:
a) 2/3 b) 4/3 c) 3/4 d) 16/9
2) if a: b = 5 : 3, then (5a + 8b): (6 a - 7b) is equals to:
a) 40 : 9 b) 9 : 49 c) 49 : 9 d) 25: 9
3) The fourth proportional to 7, 13 and 35 is:
a) 65 b) 62 c) 52 d) 50
4) The third proportional to 9 and 15 is:
a) 10 b) 15 c) 18 d) 25
5) if (7m + 2n)/(7m - 2n)= 5/3 then m: n is:
a) 7 : 8 b) 2:7 c) 8 : 7 d) 1:8
6) The mean proportion between 28 and 63 is:
a) 42 b) 45 c) 36 d) 32
7) if fourth proportional to 3,12, 15 is:
a) 40 b) 45 c) 60 d) 62
8) If x : y= 2:3 then (3x +2y)/(2x + 5y) is:
a) 12/19 b) 19/12 c) 12/13 d) 19/21
9) The mean proportion between x-y and x³ - x²y is :
a) x(x + y) b) x²(x - y) c) x²(x + y) d) x(x - y)
10) If a :b = 2: 3 and b: c= 4:5, then a: c is
a) 12 :15 b) 15:7 c) 15:8 d) 8:15
11) If a/b = c/d, then (a+ c)/(b+ d) is equal to
a) a/b b) c/d c) both a and b d) neither a nor b
12) The fourth proportional to 3, 6 and 4.5 is
a) 10 b) 9 c) 8.5 d) 6
13) The third proportional to x - y and x²- y² is
a) (x + y)(x²- y²) b) (x - y)(x² +y²)
c) (x² + y²)(x²- y²) d) (x + y)(x- y)
14) if a/b : c/d, then each ratio is equals to :
a) a+ b : c+ d b) a+ c : b + d c) a+ d: b + c d) a- b: c - d
15) the mean proportion between a²b and 1/b is
a) a b) a² c) ab d) √(ab)
16) If 2x = 3y and 4y= 5z, then 8x/z is equals to
a) 5 b) 15 c) 10 d) 8
17) Two numbers are in the ratio 1 :4. If the mean proportion between them is 28 and third proportional to them is 224, then the smaller number is
a) 12 b) 14 c) 16 d) 21
18) if three quantities a, b, c are continued proportion, then the mean proportion between
a) a and c is b b) b and c is a c) c and a is b d) all the above are true
19) x, y, z are in continue proportion, then x/z is equals to
a) y²/z² b) y²/x² c) x²y² d) x/y²
20) If (4a + 9b)/(4c + 9d) = (4a - 9b)/(4c - 9d), then a: b=
a) c: d b) d: c c) c: d+ c d) d: c - d
1b 2c 3a 4d 5c 6a 7c 8a 9d 10d 11c 12b 13a 14b 15a 16b 17b 18a 19a 20a
SHORT QUESTIONS
1) If (3a + 2b) : (5a + 3b)= 18: 29, find a, b.
2) What least number must be added to each of the numbers 2, 5, 18 and 33, so that the resulting numbers are proportional .
3) If b is the mean proportiona between a and c, show that b(a+ c) is the mean proportion between (a²+ b²) and (b²+ c²).
4) If a: b = c : d, then prove that (a+ b): (c + d)= √(a²+ b²): √(c²+ d²).
5) If x/(b - c) = y/(c - a)= z/(a - b), then show that ax+ by + cz =0.
6) If a, b, c, d are in continued proportion, prove that (b + c)(b + d)= (c + a)(c + d).
7) it a/b = c/d, then, show that (3a - 5b)/(3a + 5b)= (3c - 5d)/(3c + 5d).
1) 4:3 2) 2
LONG ANSWER
1) If b is the mean proportion between a and c, show (a²+ b²+ c²)/(a⁻² + b⁻² + c⁻²) = b⁴.
2) if a, b, c are in continued proportion, show that (2a²+ 7b²- 5ab)/(2b²+ 7c²- 5bc)= a/c.
3) If a, b, c, d are in continued proportion, show that
√(ab) + √(bc) - √(CD)= √(a+ b - c)(b + c - d).
4) If a: b= c : d, show that (a²+ c²+ ac): (a²+ c²- ac)= (b²+ d²+ bd):(b²+ d²- bd)
5) If ax = by = cz, then show that x²/yz + y²/zx + z²/xy = bc/a²+ ca/b²+ ab/c².
6) If x= {√(a+ 3b) +√(a - 3b)}/{√(a+ 3b)- √(a - 3b)}, show that 3bx²- 2ax + 3b = 0
7) If p= 4xy/(x + y), show that (p+ 2x)/(p- 2x) + (p+ 2y)/(p - 2y)= 2.
8) If If {√(a+ 15) +√(a - 6)}/{√(a+ 15)- √(a - 6)}= 7/3, find the value of a.
9) If 11 = {√(6x)+√(3x +7)}/{√(6x)- √(3x+7)}, then find x
8) x=10 9) x=6
REMAINDER THEOREM AND FACTOR THEOREM
1) (x+1) Is a factor of
a) x³+ 4x²+ 5x +2 b) x³- 4x²- 5x +2 c) x³- 3x²- 7x -1 d) 2x³- 4x²- 5x +1
2) On dividing mx³+ 9x²+ 4x -10 by (x +3), the remainder is 5. The value of m is
a) -1 b) 2 c) -2 d) 3
3) When x⁴+1 is divided (x +1), the remainder is
a) 0 b) 2 c) 1 d) -1
4) if a polynomial p(x) is divided by (x + k), then the remainder is
a) p(k) b) p(-k) c) kp(x) d) p(x)+ k
5) The remainder when x⁴- x³+ x² - x +1 is divided by (x -1) is
a) 0 b) 1 c) -1 d) 2
6) if (x -2) is a factor x²+ mx - 2, then the value of m is
a) 1 b) -1 c) 0 d) -2
7) when f(x) is divided by (ax - b), then the remainder is:
a) f(a) b) f(ab) c) f(-b/a) d) f(b/a)
8) when a polynomial f(x) is divided by (3x +4), the remainder is
a) f(3/4) b) f(4/3) c) f(-3/4) d) f(-4/3)
9) if (x -2) is a factor of x² - 4x +m, then the value of m is
a) -4 b) 4 c) 0 d) -1
10) If (x -1) is a factor of x³+ 2x²- x +k, then the value of k is
a) 1 b) 2 c) -2 d) -1
11) if (2x -1) is a factor of f(x)= 2x²+ px -5, then the value of p is
a) 10 b) 9 c) 8 d) 5
12) If (x- 2) is a factor of f(x)= x³+ kx²- 5x -6, then the value of k is
a) 2 b) 1 c) -1 d) -2
13) Given f(x)= 3x²- 5x +p. If (x -2) is a factor of f(x), then the value of p is
a) -2 b) 2 c) -1 d) 1
14) If (x -1) and (x +2) are factors of f(x)= x³+ 10x²+ ax +b, then the value of b is
a) 10 b) 12 c) 18 d) -18
15) When the polynomials f(x)= px³+ 3x²-3 and g(x)= 2x³-5x + p are divided by x - 4 , they leave the same reminder. The value of p is
a) -1 b) 0 c) 1 d) 2
16) Given f(x)= x³+ (kx+8)x +k. If f(x) is divided by x + 1 , the remainder is
a) 2k- 9 b) 2k+9 c) 2k d) 0
17) If (x -3) is a factor of f(x)= x² - kx + 12, then the other factor of f(x) is
a) x- 4 b) x -1 c) x - 2 d) x+ 4
18) (x +2) and (x -3) are factors of f(x)= x³+ ax +b , then the value of f(-3) is
a) 1 b) 2 c) -1 c) 0
19) Given f(x)= (3km+2)x³+ (k -1). If f(x) is divided by (2x+1), the remainder is
a) f(1/2) b) f(-1/2) c) f(2) d) f(-2)
20) On dividing x²- 4x +m by x-2, the remainder is -1. The value of m is
a) 1 b) 2 c) -2 d) 3
1a 2b 3b 4b 5b 6b 7d 8d 9b 10c 11b 12a 13a 14d 15c 16a 17a 18d 19b 20d
SHORT QUESTIONS
1) Using reminder theory, find the value of k if on the dividing 2x³+ 3x² - kx +5 by (x -2), leaves a remainder 7.
2) What must be subtracted from 16x³- 8x²+ 4x +7 so that the resulting expression has 2x + 1 as a factor ?
3) When divided by x -3, the polynomials p(x)= x³ - px²+ x + 6 and Q(x)= 2x³- x²-(p+3)x -6 leave the same remainder. Find the value of p. Also find the remainder .
4) Find the value of k, if x - 2 is a factor of x³+ 2x²- kx + 10. Hence, determine whether x + 5 is also a factor.
5) Find a, if the two polynomials ax³+ 3x²-9 and 2x³+ 4x+ a leave the same remainder when divided by x + 3. also find the remainder.
6) if p(x)= 2x³+ 3x² - ax - b is divided by x - 1, the remainder is 6, then find the value of a + b.
7) Find the value of p if x³+ px +2p -2 is exactly divisible by x + 1.
1) 13 2) 1 3) 1,27 4) 13, yes 5) 3, -63 6) -1 7) 3
LONG QUESTION
1) If x + 2 and x+ 3 are factors of x³+ ax +b, find the value of a and b.
2) Use factor theorem to factorise 6x³+ 17x²+ 4x -12 completely.
3) If x- 2 is a factor of 2x³ - x²- px - 2,
a) find the value of o
b) with the value of p, Factorize the above expression completely .
4) Given that (x +2) and (x +3) are factors of 2x³+ ax²+ 7x - b, determine the value of a and b.
5) Using reminder theorem, factorise completely the following polynomials: 3x³+ 2x²- 19x +6
6) if x - 2 is a factor of the expression, 2x³+ ax²+ bx -14 and when the expression is divided by x-3, it leaves a remainder 52, find the value of a and b.
7) Using remainder theorem and factor theorem, factorise the following polynomial : x³+ 10x²- 37x +26.
1) -19,-30 2) (x +2)(2x +3)(3x -2) 3) 5, (x -2)(x +1)(2x +1) 4) 9,6 5) (x -2)(x +3)(3x -1) 6) 5, -11 7) (x -1)(x +13)(x -2)
MATRIX
1) Which of the following is a row matrix ?
a) matrix P of order 2x2
b) matrix Q of order 1x2
c) Matrix R of order 2x1
d) Matrix S of order 3x2
2) The order of matrix is 2x3. It has:
a) 5 element b) 6 elements c) 1 elements d) none
3) If A= 4 -2 B= 3 5 & C= 1 3
5 7 -4 -2 -2 4 , then the value of A+ B C is
a) 6 1 b) 6 0 c) 6 3 d) 6 6
3 0 3 1 0 1 3 1
4) If A= 4 x & B= y -3 C= 10 0 with the relation 3A+ 2B = C, then:
a) x=1, y=0 b) x=-1, y=0 c) x=2, y= -1 d) x=-2, y=1
5) Order of matrix A is 2x3 and the order of matrix B is 3x1. The order the matrix AB is:
a) 2x1 b) 1x 2 c) 3 x 1 d) 2x 3
6) Which of the following is a diagonal Matrix ?
a) 0 6 1 b) 2 0 0
2 0 4 0 -1 0
1 8 0 0 0 -2
c) 4 0 0 d) 1. 0 0
0 -2 0 0 1 1
0 0 1
7) In a null matrix:
a) all the elements are 0
b) every diagonal element is zero
c) every non-diagonal element is zero
d) every element is one.
8) 1 8 9 is a:
a) row matrix b) column matrix c) diagonal matrix d) null matrix
9) The transpose of matrix
2 -1 4
1 7 3
-4 1 5 is:
a) 2 1 4 b) 2 4 -1
-1 1 5 1 3 7
4 7 3 -4 5 1
c) -1 4 2 d) 2 1 -4
7 3 1 -1 7 1
1 5 -4 4 3 5
10) The order of matrix A is 2x 3 and that of B is 3x1.
a) AB is possible, but BA is not possible.
b) BA is possible but AB is not possible
c) AB as well as BA are not possible
d) AB as well as BA are possible
11) if A= 2 -4 & B= 1 -1
0 1 0 5, then the value of B+ A is
a) 3 -5 b) 6 2 c) 1 -2 d) -3 5
0 6 -1 4 -4 1 0 -6
12) If A= 2 5 B= 1 -1
1 3 -3 2 then AB is equal to
a) -13 5 b) 8 -1 c) -13 8 d) -1 0
1 8 5 2 -8 5 7 -2
13) If A= 3 -4 &B= -1 2
5 6 5 7 then the order of 3A - 2B is
a) 2x1 b) 4x4 c) 1x1 d) 2x2
14) If A= 2 5 & B= 1 -3
-3 7 2 5 then B - A is equal to
a) 4 -1 b) -1 -8 c) -1 8 d) -2 4
6 -2 5 -2 5 4 -1 -2
15) If M= 1 -2 & N= 2 1
-1 2 then the order of MN is
a) 1x2 b) 2x1 c) 1x1 d) 2x2
16) If A= -2 3 B= 5 2
4 5 -7 3 then transpose of matrix (A+ B) is:
a) 3 5 b) 3 -3 c) 3 8 d) 3 5
-3 8 5 8 -3 5 -8 3
17) If A= 2 1 & B= 5 -1
-1 4 2 -1 then Aᵗ - Bᵗ is equal to:
a) 1 -1 b) 7 -1 c) -3. -1 d) -3 -3
4 2 2 5 2 4 2 5
18) If A= 1 2 B= x. 0
3 3 0 y then the order of the matrix BA is
a) 1x2 b) 2x1 c) 2x3 d) 2x2
19) If A= 2 0 B= 0 1
-3 1 -2 3 then the matrix BA is
a) -3 1 b) -4 5 c) -3 2 d) 4 -1
-13 3 -2 7 5 -7 2 19
20) If A= x 3 & A²= 3I, where I= 1 0
y 3. 0 1 the order of A² is
a) 2x2 b) 2x3 c) 1x2 d) 3x2
1b 2b 3b 4c 5a 6b 7a 8a 9d 10a 11a 12c 13d 14b 15a 16b 17d 18d 19a 20a
Short Answer type Questions
1) If A= 3 -1
0 2 find matrix B such that A²- 2B = 3A + 5I, where I is 2x2 identity matrix.
2) Given matrix
A= 4sin30° cos0° & B= 4
Cos0° 4sin30° 5 if AX = B
a) Write the order of matrix X.
b) Find the matrix X.
3) Find the value of x and y if
A= x 7 B= 6 -7 C= 10 7
9 y-5 4 5 22 15 with the relation 2A+ B = C
4) Simplify:
A= sinA -cosA & B= cosA sinA
CosA sinA -sinA cosA with the relation A sinA+ B cosA
5) A= x 3
y 3 if A²= 3I where I is the identity matrix of order 2, find x and y
6) A= 1 4 & B= 3 2
-2. 3. 0 -3 with the relation A+ 2M = 3B, find the matrix M
7) If A= p 0 B= 0 -q C= 2 -2
0 2 1 0 2 2 and BC = C², find the values of p and q
8) If A= 2x x B= 3 & C= 16
y 3y 2 9 with the relation AB = C then find x and y
9) If A= 3 -2 B= 6 C= -4 D= 2
-1 4 1 5 2 find the value of AB+ 2C - 4D.
10) Evaluate:
A= 4sin30 2cos60 B= 4 5
Sin90 2 cos0 5 4 find the value of AB
1) -5/2 -1
0 -7/2
2) 2x1 1
2
3) 2,10
4) 1 0
0 1
5) -3,-2
6) 4 1
1 -6
7) 8,4
8) 2,1
9) 0
0
10) 13 14
14 13
LONG ANSWER TYPE QUESTIONS
1) If A= 2 5 B= 4 -2
1 3 -1 3 and I is the identity matrix of the same order and A' is the transpose of matrix A, find A'B + BI.
2) Let A= 2 1 B=4 1 & C=-3 2
0 -2. -3 -2 -1 4 find A²+ AC - 5B
3) A= 2 0 I= 1 0
-1 7 0 1 and A²= 9A + mI. Find m.
4) If A= 1 3 B= -2 1
3 4 -3 2 and A²- 5B²= 5C, find matrix C, where C is a 2 by 2 matrix.
5) B= 1 1
8 3 find the matrix X if X= B²- 4B. Hence solve for a and b given
X= a & C= 5
b 50 with X= C
6) If A= 2 3 B= 0 4 C= 1 0
5 7 -1 7 -1 4, find AC + B²- 10C.
7) If A= 4 2
-1 1 with relation AM=6I where M is a matrix and I is unit matrix of order 2x2.
a) State the order of oM
b) Find the matrix M.
8) A= 3 0 B= -4 2
5 1 1 0 find A²- 2AB + B².
1) 11 -3
16 2
2) -23 3
17 6
3) -14
4) 1 3
3 4
5) 5 0
0 5 1, 10
6) -15 40
1 33
7) 2x2 1 -2
1 4
8) 51 -20
54 -17
ARITHMETIC PROGRESSION
1) The mth term of the AP 5, 11, 17, 23,.... is
a) 6m - 1 b) 6m +1 c) 5m -1 d) 6n -1
2) If nth term of an AP is 2n-1, then its 20th term is
a) 33 b) 34 c) 36 d) 39
3) The number of terms in the AP 7, 16, 25,.... 349 is
a) 40 b) 39 c) 38 d) 35
4) The sum of first 8 multiples of 3 is
a) 108 b) 100 c) 96 d) 95
5) If the nth term of an AP is Tₙ= 5 - 3n, then its common difference is
a) -2 b) -3 c) 3 d) 4
6) The first term of the AP whose pth term is 3p - 1 is
a) 2 b) 3 c) 4 d) 5
7) The 10th term of the AP 120, 116, 112 ....is
a) 80 b) 82 c) 84 d) 90
8) The common difference of the AP - 15, - 12, - 9, .... is
a) 3 b) - 3 c) 2 d) - 2
9) if the sum of the first n terms of an AP is given by Sₙ= -5n +1. The common difference of the AP is
a) -8 b) 8 c) 7 d) -1
10) The nth term of an AP is given by Tₙ = -5n +1. The common difference of the AP whose is
a) -5 b) -4 c) 4 d) -2
11) For an AP, if a= 5, d= 3 and tₙ = 50, then the value of n is :
a) 10 b) 16 c) 20 d) 21
12) The sum of the first 6 multiple of 5 is
a) 100 b) 102 c) 104 d) 105
13) Which term of the AP 4, 9, 14, 19.... is 124 ?
a) 20th b) 22nd c) 24 th d) 25th
14) The 10th term from the end of the AP -2, -6, -10,....-110 is
a) -72 b) 74 c) -74 d) -70
15) If (5m+2), (4m -1) and (m +2) are in AP, then the value of m is
a) 1 b) 2 c) -1 d) 3
16) The 11th term of the AP -3, -1/2, 2.... is
a) 22 b) 21 c) 20 d) 16
17) The common difference of the AP whose pth term is 8p+1, is
a) 1 b) 4 c) -7 d) 8
18) The nth term of the AP 2, 5, 8, ..... is
a) 3n+1 b) 2n -1 c) 2n+3 d) 3n -1
19) The 100th term of the AP x, x+1, x+2,.... is
a) 99 b) x c) x + 99 d) x - 99
20) The 6th term from the end of the AP 17, 14, 11,.... -40 is
a) 25 b) -25 c) -20 d) 40
1a 2d 3b 4a 5b 6a 7c 8a 9c 10a 11b 12d 13d 14c 15d 16a 17d 18d 19c 20b
SHORT QUESTIONS
1) Find the 16th term of the AP 7, 11, 15, 19 ..... Also find the sum of the first 6 terms.
2) The 2nd and 45th term of an arithmetic progression are 10 and 96 respectively . Find the first term and the common difference and hence find the sum of the first 15 terms.
3) if the 6th term of an AP is equal to four times its first term and the sum of first six term is 75, find the first term and the common difference.
4) Which term of the AP 3, 15, 27, 39...... will be 120 more than 21st term?
5) For what value of n, are the nth terms of two AP's 63, 65, 67,.... and 3, 10,17,... equal ?
6) Find the 20th term from the end of the AP 3, 8, 13,..... 253.
7) The first and last term of an AP are 5 and 45. If the sum of the terms is 400, find the number of the terms and the common difference.
8) Find the sum of the first 17 terms of an AP whose 4th, and 9th terms are -15 and -30 respectively.
9) Find the sum of odd numbers between 0 and 50.
1) 67,102 2) 8,2,330 3) 5,3 4) 31st 5) 13 6) 158 7) 16, 8/3 8) -510
LONG QUESTIONS
1) In an arithmetic progression (AP) the 4th and 6th terms are 8 and 14 respectively. Find the
a) first term
b) common difference
c) sum of the first 20 terms.
2) Find the value of the middle most term/s of the AP -11, -7, -3,....49.
3) If m times the mth term of an AP is equal to n times the nth term show that (m + n)th term of the AP is 9.
4) If the sum of first 7 terms of an AP is 49 and that of the 17 term is 289, find the sum of the first n terms .
5) The sum of the first 16 terms of an AP is 112 and the sum of its next 14 terms is 518. Find the AP.
6) If the first term of an AP is 2 and the sum of the first five terms is equal to one fourth of the sum of the next five terms, then
a) show that t₂₀ = 112
b) find the sum of first 30 terms.
7) How many terms of the AP 17,15,13..... must be added to get the sum 72? Explain the double answer.
8) If the pth, qth, and rth terms of an AP be a, b and c respectively, then show that a(q - r)+ b(r - p)+ c(p - q)= 0
1) -1,3,550 2) 17,21 4) n² 5) -8,-6,-4 6) -2550 7) 6 or 12
GEOMETRIC PROGRESSION
1) A list of numbers in which each term is obtained by____ its preceding term by a fixed (non zero) number, except the first term, is called a geometric progression.
a) adding b) multipleing c) subtracting d) dividing
2) The least members a₁, a₂, a₃.... forms a GP, if and only if _____= r, a fixed number.
a) aₙ₊₁/aₙ b) aₙ/aₙ₊₁ c) aₙ₋₁/aₙ d) all of these
3) If r, ar, ar²,.... is a GP, then its general term is denoted by Tₙ=
a) arⁿ b) arⁿ⁺¹ c) arⁿ⁻¹ d) ar
4) If a finite GP a, ar, ar²,.... contains n terms and its last term is l, then l=
a) a/rⁿ⁻¹ b) arⁿ⁻¹ c) arⁿ⁺¹ d) arⁿ
5) If a, ar, ar²,.... is a finite GP consisting of m terms, then nth term from end is
a) arᵐ⁻ⁿ b) arᵐ c) arᵐ⁺ⁿ d) arᵐ⁺ⁿ⁻²
6) The numbers a, b, c are in GP if
a) a²= bc b) b²= ac c) c²= ab d) a- b = b - c
7) If a, ar, ar²,.... is a finite GP with last term l, then nth term from end=
a) (1/r)ⁿ⁻¹ b) l(1/r)ⁿ c) l(1/r)ⁿ⁻¹ d) lrⁿ⁻¹
8) If the product of numbers in GP is given, then four numbers are taken as___ respectively.
a) ar², ar³, ar, a/r b) a/r², a/r, ar, ar³ c) a/r³, a/r, ar, ar³ d) a/r², a/r, ar, ar²
9) the list of numbers 1/9, -1/3, 1, -3.... is a GP with r=
a) -3 b) -1/3 c) 3 d) 1/3
10) The 11th term of the GP 1/8, -1/4, 2, -1,.... is
a) 64 b) -64 c) 128 d) 1024
11) The 5th term from the end of the GP 2, 6, 18,... 13122 is
a) 162 b) 486 c) 54 d) 1458
12) If k, 2(k +1), 3(k +1) are three consecutive terms of a GP, then the value of k is
a) -1 b) - 4 c) 1 d) 4
13) ____ term of the GP 18, 12,8, ...is 512/729
a) 12th b) 10th c) 9th d) 11th
14) if x, 2y, 3z are in AP where the distinct numbers x, y, z are in GP, then the common ratio of the GP is
a) 3 b) 1/3 c) -3 d) none
15) if the first and the nth terms of a GP are a, b respectively, and if p is the product of n terms, then p²=
a) ab b) abⁿ c) (ab)ⁿ d) none
16) If a is the first term and r is the common ratio of a GP then, Sₙ= ____, where r≠ 1.
a) a(1- rⁿ⁻¹)/(1- r) b) a(1- rⁿ)/(1- r) c) a(1- ⁿ⁺¹)/(1- r) d) arⁿ⁻¹
17) If l is the last term of a GP with a as the first term and r as the common ratio, then Sₙ= ____, r≠ 1.
a) (a + lr)/(1+ r) b) (a - lr)/(1+ r) c) (a - lr)/(1- r) d) (a + lr)/(1- r²)
18) The sum of the first five terms of the list of numbers 3, 6, 12,... is
a) 93 b) 31 c) 96 d) 33
19) The sum of the first 8 terms of the series 1 + √3 + 3 + ....is
a) 40(√3-1) b) 40(√3+1) c) 80(√3-1) d) 40/(√3+1)
20) The sum of the first 6 terms of the GP 1, -2/3, -4/9.... is
a) -133/243 b) 133/243 c) 793/1215 d) 667/1215
21) if the sum of the GP 1, 4, 16,.....is 341, then the number of terms in the GP is
a) 8 b) 6 c) 5 d) 10
22) ____ terms of the GP 3, 3/2, 3/4,..... are needed to give the sum 30169/512.
a) 9 b) 10 c) 11 d) 12
23) Given a GP with a= 729 and 7th term as 64, then S₇=
a) 2059 b) 462 c) -2016 d) 2060
24) ¹¹∑ₖ₌₁(2+ 3ᵏ) =
a) (41+ 3¹⁰)/2 b) (41+ 3¹²)/2 c) 41+ 3¹² d) (44+ 3¹²)/2
1b 2d 3c 4b 5a 6b 7c 8c 9a 10c 11a 12b 13c 14b 15c 16b 17c 18a 19b 20b 21c 22b 23a 24b
SHORT QUESTIONS
1) Which term of the GP 5, 10, 20,..... is 20480 ?
2) Find the 6 term from the end of the GP 8, 4, 2, 1, 1/2,.....1/1024.
3) if the 4th and 7th terms of a GP are 1/18 and -1/486 respectively , then find the GP.
4) The 4th, 9th and last terms of a GP are 10, 320 and 2560 respectively, find the first term and the number of terms in the GP.
5) The third term of a GP is 4. Find the product of its first terms.
6) The first term of a GP is 1. The sum of the third and 5th terms is 90. Find the common ratio of the GP.
7) Find the sum: 243+ 324 + 432+.... up to n terms.
8) Determine the number of terms n in the GP T₁ , T₂....Tₙ, if T₁= 3, Tₙ= 96 and Sₙ = 189.
9) How many terms of the GP 1, 4, 16, 64.... will make their sum 5461 ?
10) The sum of n terms of the progression is 3ⁿ -1. Show that it is a GP. Find its common ratio .
11) The first term of a GP is 27 and its 8th term is 1/81. Find the sum of its first 10 terms.
1) 13 2) 1/32 3) -3/2,1/2,-1/6,1/18...... 4) 5/4,12 6) ±3 7) (3⁶/3ⁿ) (4ⁿ - 3ⁿ) 8) 6 9) 7 10) 3 11) (81/2)(1- 1/3¹⁰)
LONG ANSWER TYPE QUESTIONS
1) The sum of three numbers which are constitutive terms of an AP is 21. If the second number is reduced by 1, and the 3rd is increased by 1, we obtained three consecutive terms of a GP. Find the numbers.
2) The sum of three numbers in GP is 38 and their product is 1728. Find the numbers.
3) Find the least value of n for which the sum 1+ 3 + 3²+.... to n terms is greater than 7000.
4) Find the sum to n terms of the series whose nth term is 2ⁿ + 3n.
5) Find the sum of the following to n term 7+ 77 +777+.....
1) 12,7,2 or 3,7,11 b) 8,12,18 or 18,12,8 3) 9 4) {4(2ⁿ-1)+ 3n²+ 3n}/2 5) (7/81)(10ⁿ⁺¹ - 9n -10)
REFLECTION
1) Which of the following points is invariant with respect to the line to y=- 2?
a) (2,2) b) (3,-2) c) (-2,3) d) -2,2)
2) Which of the following points is invariant with respect to the line x= 4 ?
a) (0,4) b) (-4,0) c) (0,-4) d) (4,2)
3) The reflection of the point (1,-5) in the origin is the point.
a) (1,5) b) (- 1,5) c) (-1,5) d) -5,1)
4) The reflection of the point (4,-2) in the line x=2 is
a) (0,0) b) (-4,2) c) (2,-2 0) d) (0,2)
5) The reflection of the point (4,-2) in the line y= -1 is
a) (4,0) b) (4,2) c) (4,-3) d) (0,4)
6) If the image of the point P under reflection in the x-axis is (-2,6), then coordinanates of point P are.
a)) (2,-6) b) (-2,6) c) (-2,-6) d) (2,6)
7) The reflection of the point (0,-1) in the y-axis is
a) (0,-1) b) (0,1) c) (- 1,0) d) (0,0)
8) The reflection of the point (-2,4) in the x-axis is
a) (2,4) b) ( 2,-4) c) ( 4,-2) d) (-2,-4)
9) The reflection of the point (-5,0) in the x-axis is
a) (5,0) b) (0,5) c) (-5,0) d) (0,-5)
10) Which of the following points is invariant with respect to the line y= -2?
a) (3,4) b) (3,-4) c) (4,3) d) (-4,2)
11) The reflection of the point (-4,0) in the origin is
a) (4,0) b) (-4,0) c) (0,-4) d) (0,4)
12) The reflection of a point P in the y-axis is P'(-4,-2). The coordinanates of point P are
a) (-4,2) b) (4,2) c) (4,-2) d) (-2,4)
13) Point (0,-2) is invariant under reflection in:
a) xaxrb) y-axis c) orird) none
14) Point (5,0) is invariant under reflection in
a) x-axis b) y-axis c) origin d) none
15) Point P is first reflected in x-axis to P'. P' is then reflected and y-axis to P"(-2,5). The coordinates of P are
a) (2,5) b) (-2,-5) c) (2,-5) d) (-5,2)
1b 2d 3c 4d 5a 6c 7a 8d 9c 10a 11a 12c 13b 14a 15c
SHORT ANSWER TYPE QUESTIONS
1) The point P (a,b) is first refleted in the origin and then reflected in the y-axis to P'. if P' has coordinate (3,-4), evaluate a, b.
2) The point A(-6,4) on reflection in y-axis is mapped as A'. Point A' on reflection in the origin is mapped as A".
a) Find the co-ordinates of A'.
b) Find the coordinates of A".
c) Write down a single transformation that maps A to A".
3) a) point P (a, b) is reflected in x-axis to P'(4,-3). Write down the value of a and b.
b) P" is the image of P when reflected in y-axis. Write down the coordinates of P".
c) Name a single transformation that maps P' to P".
4) A triangle with the vertices A(1,2), B(4,4) and C(3,7) is first reflected in the line y= 0 onto ∆A'B'C' and then ∆A'B'C' is reflected in the origin onto ∆A"B"C". Write down the coordinates of
a) A', B' and C'
b) A", B" , C".
5) Write down the coordinates of the image of the point (3,-2) when :
a) reflected in x-axis.
b) reflected in y-axis.
c) reflected in the origin.
1) 3,4
2) (6,4) (-6,-4) Rₓ
3) (4,3) (-4,3) Rₒ
4) (1,-2),(4,-4),(3,-7) (-1,2),(-4,4),(-3,7)
5) (3,2) (-3,-2) (-3,2)
LONG ANSWER TYPE QUESTIONS
1) Plot the point A(2,-3), B(-1,2) and C(0,2) on the graph paper. Draw the triangle formed by reflecting these points in the x-axis. Are the two triangles congruent ?
2) The point (3,0) and (-1,0) are invariant points under reflection in the line L₁, while the points (0,-3) and (0,1) are invariant points under reflection in the line L₂.
a) Name the lines L₁ and L₂.
b) Write down the images of the points P(3,4) and Q(-5,-2) on reflection in L₁. Name the images as P' and Q' respectively.
c) Write down the images of P and Q on reflection in L₂. Name the images as P" and Q" respectively.
d) State or describe single transformation that maps P' onto P".
3) Plot the points A(-2,0), B(4,0), C(1, 4) and D(-2,4) on a graph paper. Point D is reflected about the line x= 1 to get the image E. Write the coordinates of E. Name the figure ABED.
4) Use graph paper for this question:
a) The plot P(2,3) is reflected in the line x= 4 to the point P'. Write down the coordinates of P '.
b) Find the image of the point Q(1,-2) in the line x= -1.
5) Use graph paper for this question
a) Find the Co-ordinates of the images of (3,1) under reflection in x-axis followed by reflection in the line x= 1.
6) Use graph paper for this question: Points A and B have coordinanates (2,5) and (0,3) respectively. Find
a) the image A' of A under reflection in x-axis.
b) the image B' of B under reflection in the line AA'.
1) yes
2) x-axis, y-axis (3,-4),(-5,2) (-3,4),(5,-2) Rₒ
3) (4,4), rectangle
4) (6,3) (-3,-2)
5) (-1,-1)
6) (-2,-5) (4,3)
LONG ANSWER TYPE QUESTIONS - II
1) Use graph paper for this question
a) Plot the points A(4,6) and B (1,2)
b) A' is the image of A when reflected in x-axis.
c) B' is the image of B when B is reflected in the line AA'.
d) Give the geometrical name for the figure ABA'B'.
2) Use graph paper for this question:
A(0,3), B(3,-2) and O(0,0) are the vertices of triangle ABO.
a) plot the triangle on the graph papers taking 2cm = 1 unit on both the axis .
b) plot D, the reflection of B in the y-axis, and write its coordinates.
c) Give the geometrical name of the figure ABOD.
3) Use graph paper to answer the following questions. (Take 1cm = 1 unit on both axes)
a) Plot A(4,4), B(4,-6) and C(8,0), the vertices of a triangle ABC.
b) Reflect ABC on the y-axis and name it as A'B'C'.
c) Write the coordinanates of the image A', B' and C'.
d) Give a geometrical name for the figure AA'C'B'BC.
4) Use graph paper and take 1cm= 1unit along both x-axis and y-axis.
a) Plot the point A(-4,4) and B(2,2).
b) reflect A and B in the origin to get the image A' and B' respectively.
c) Write down the coordinate of A' and B'.
d) Give the geometrical name for the figure ABA'B'.
5) Using a graph paper, plot the points A(6,4) and B(0,4).
a) Reflect A and B in the origin to get the image A' and B'.
b) Write the coordinates of A' and B'.
c) State the geometrical name for the figure ABA'B'.
d) Find its perimeter.
6) Use graph paper to answer the following questions. (Take 2cm= 1 unit on both axis).
a) plot the points A (-4,2) and B (2,4).
b) A' is the image of A when reflected in the y-axis. Plot it on the graph paper and write the coordinates of A'.
c) B' is the image of B when reflected in the line AA'. Write the coordinates of B'.
d) Write the geometric name of the figure ABA'B.
7) Use graph paper for this question taking 1cm= 1 unit along both the x and y axis.
a) plot the pointa A(0,5), B(2,5), C( 5,2), D( 5,-2), E( 2,-5) and F(0,-5).
b) Reflect the points B, C, D, E on the y-axis and name them respectively as B', C', D', E'.
c) Write the coordinates of B', C', D', E'?
d) Name the figure formed by BCDEE'D'C'B'.
1) kite
2) kite
3) (-4,4),(-4,-6),(-8,0) hexagon
4) (4,-4),(-2,-2) rhombus
5) (-6,-4),(0,4) parallelogram 32 units
6) (4,2) (2,0) kite
7) (-2,5),(-5,2),(-5,-2),(-2,-5) regular octagon
SECTION AND MIDPOINT FORMULA
1) The centroid of the triangle whose vertices are (3,7),(-8,6) and (5,10) is
a) (0,9) b) (0,3) c) (1,3) d) (3,3)
2) A line interestc the y-axis and x-axis at the points A and B respectively. If (2,-5) is the midpoint of AB, then the coordinates of A and B are, respectively :
a) (0,-5) and (2,0) b) (0,10) and (-4,0) c) (0,4) and (-10,0) d) (0,-10) and (4,0)
3) If O (a/3,4) is the midpoint of a line segment joining the point X(-6,5) and Y(-2,3), then the value of a is
a) -4 b) -6 c) 12 d) -12
4) If the centroid of the Triangle formed by (7,x),( y,- 6) and (9,10) is (6,3), then the values of x and y respectively are:
a)( 5,3) b) ( 5,2) c) (-3,2) d) (6,5)
5) The ratio in which the point (3/4, 5/12) divides the line segment joining the point A(1/2, 3/2) and (2,-5) is
a) 1:2 b) 3 : 2 c) 1:5 d) 2 :3
6) The fourth vertex D of a parallelogram ABCD whose three vertices are A(-2,3), B(6,7) and C(8,3) is
a) (0,1) b) (0,-1) c) (-1,0) d) (1,0)
7) The ratio in which the point P(4, m) divides the line segment joining the points A(2,3) and B(6,-3) is
a) 1:2 b) 2:1 c) 1:3 d) 1:1
8) If A(m/2,5) is the midpoint of the line segment joining the points Q(-6,7) and R(-2,3), then the value of m is
a) -8 b) -4 c) 12 d) 6
9) The midpoint of the line segment joining the points(-5,7) and (-1,3) is
a) (-3,7) b) (- 3,5) c) (- 1,5) d) ( 5,-3)
a) (6,9) b) (7,9) c) (-7,9) d) (7,-9)
11) The ratio in which P(m,4) divides the line segment joining the points A(2,5) and B(6,-3) is
a) 1:2 b) 2: 1 c) 1:3 d) 1 :7
12) if the midpoint of the line segment joining the points P(6, b - 2) and Q(- 2,4) is (2,-3), then the value of b=
a) -5 b) -6 c) -7 d) - 8
13) If the coordinates of one end of a diameter of a circle are (2,3) and the coordinates of its centre are (-2,5), then the co-ordinates of the other end of the diameters are:
a) (-6,7) b) (6,-7) c) (6,7) d) (-6,-7)
14) The point which lies on the perpendicular bisector of the line segment joining the points A(-2,-5) and B(2,5) is
a) (0,0) b) (0,2) c) (2,0) d) (-2,0)
15) The vertices of a parallelogram in order are A(1,2), B(4, y), C(x, 6), D(3,6). The value of x and y respectively are:
a) 6, 2 b) 3, 6 c) 5, 6 d) 1,4
16) If A(1,3), B(-1,2), C(2,5) and D(x, y) are the vertices of a parallelogram ABCD, then the value of x is
a) 3 b) 4 c) 0 d) 3/2
1b 2d 3d 4b 5c 6a 7d 8a 9b 10b 11d 12d 13a 14a 15a 16b
SHORT ANSWER TYPE QUESTIONS
1) Find the ratio in which the line segment joining (-2,5) and (-5,-6) divided by the line y= -3. Hence find the point of intersection .
2) P(1,-2) is a point on the line segment joining A(3,-6) and B(x, y) such that AP: PB is equal to 2:3. Find the coordinates of B.
3) In what ratio is the line segment joining P(5,3) and Q(-5,3) divided the y-axis ? Also find the coordinanates of the point of intersection.
4) In what ratio does the point C(3/5,11/5) divide the line segment joining the points A(3,5) and B (-3,-2)?
5) Find the coordinanates of the point of trisection (i.e., points dividing into three equal parts) of the line segment joining the points A(2,-2) and B(-7,4).
6) Find the ratio in which the y-axis divides the line segment joining the points (5,-6) and (-1,-4). Also, find the point of intersection.
7) If the points A(6,1), B(8,2), C(9,4) and D(p,3) are the vertices of a parallelogram, taken in order, find the value of p.
8) In the figure, line APB meets the x-axis at A and y-axis at B. P is the point (-4,2) and AP: PB= 1:2. Write down coordinanates of A and B.
1) 8:3, (-46/11,-3)
2) (-2,4)
3) 1:1, (0,3) 4) 2:3 5) (-1,0),(-4,2)
6) 5:1, (0,-13/3)
7) 7
8) (-6,0),(0,6)
LONG ANSWER TYPE QUESTIONS
1) Find the ratio in which the point (-3, p) divides the line segment joining the points (-5,-4) and (-2,3). Hence, find the value of p.
2) If the co-ordinate the midpoints of the sides of a triangle are (1,2),(0,1) and (2,-1), find the coordinates of its vertices.
3) The base BC of an equilateral triangle ABC lies on y-axis. The co-ordinates of point C are (0,-3). If the origin is the mid point of the base BC, find the coordinates of the points A and B.
4) P and Q are the points on the line segment joining the points A(3,-1) and B(-6,5) such that AP= PQ= QB. Find the co-ordinates of P and Q.
5) Find the lengths of the median of a triangle whose vertices are A(7,-3), B(5,3) and C(3,-1).
6) The line segment joining P(-4,5) and Q(3,2) intersects the y-axis at R. PM and QN are perpendicular from P and Q on x-axis. Find
a) the ratio PR: RQ.
b) the co-ordinates of R.
c) the area of the quadrilateral PMNQ
7) The line segment joining the points (3,-4), and (1,2) is trisected at the points P and Q. If the coordinates of P and Q are (p, -2) and (5/3, q) respectively, find the value of p and q.
1) 2:1, 1,2/3
2) (1,-4),(3,2),(-1,2)
3) (0,3),(±3√3,0)
4) (0,1),(-3,3)
5) 5 units, 5 units, √10 units
6) 4:3, (0,23/7), 24.5 sq units
7) 7/3,0
EQUATION OF A STRAIGHT LINE
1) The slope of a line whose angle of inclination is 30° is
a) √3 b) 1/√3 c) -1/√3 d) -√3
2) The angle of inclination of a line having slope 1, is
a) 30° b) 45° c) 60° d) 90°
3) The slope of the line passing through the points (0,-4) and (-6,2) is
a) 0 b) 1 c) -1 d) 6
4) The slope of the line passing through the points (3,-2) and (-7,-2) is
a) 0 b) 1 c) -1 d) not defined
5) The slope of a line parallel to y-axis is
a) 0 b) 1 c) -1 d) not defined
6) The slope of a line parallel to x-axis is
a) 0 b) 1 c) -1 d) not defined
7) The slope of the line passing through the points (3,-2) and (3,-4) is
a) -2 b) 0 c) 1 d) not defined
8) The angle of inclination of the line y= x/√3 -5 is
a) 0° b) 30° c) 45° d) 60°
9) If the slope of the line passing through the points (5,2) and (3, k) is 2, then the value of k is
a) -1 b) -2 c) -3 d) - 6
10) The slope of a line parallel to the line passing through the points (6,0) and (-3,7) is
a) 7/9 b) -7/9 c) 9/7 d) - 9/7
11) The slope of a line perpendicular to the line passing through the point (2,5) and (-3,6) is
a) 5 b) - 5 c) 15 d) - 15
12) The slope of a line parallel to the line 3x + 2 y - 7 = 0 is
a) -2/3 b) 2/3 c) -3/2 d) 3/2
13) The slope of the line x - 2y = 1 is
a) 0 b) 1 c) 1/2 d) -1/2
14) The angle of inclination of the line √3 x - y - 1 = 0 is
a)n30° b) 45° c) 60° d) 90°
15) The equation of the line whose inclination is 45° and which interesects the y-axis at the point (0, - 4) is
a) x - y = 4 b) x + y = 4 c) y - x = 4 d) x - y = - 4
16) if the point (a,2a) lies on the line y= 3x -6, then the value of a is
a) 1 b) 3 c) 6 d) 7
1b 2b 3c 4a 5d 6a 7d 8b 9b 10b 11a 12c 13c 14c 15a 16c
SHORT ANSWER TYPE QUESTIONS
1) Show that the points (1,-9), (7,6) and (3,-4) are collinear.
2) The equation of a line 2√3 x -2y +5 = 0. Find
a) the slope
b) the inclination
c) y- intercept of the line.
3) if the lines 2x+ 3y= 5 and Kx - 6y = 7 are parallel then find the value of k.
4) if the lines 3x- 4y +7= 0 and 2x+ ky= - 5 are perpendicular to each other, then find the value of k.
5) Find the equation of the line whose inclination is 30° and which intersects the y-axis at the point (0,-4).
6) P(3,4), Q(7,-2) and R(-2,-1) are the vertices of ∆ PQR. Write down the equation of the median of the triangle through R.
7) Write down the equation of the line whose slope is 3/2 and which passes through the point P, where P divides the line segment joining A(-2,6) and B (3,-4) in the ratio 2:3.
8) Find the equation of a line passing through the point (-2,3) and having the x-intercept of 4 units.
9) The line 4x- 3y= -12 meets the x-axis at A. Write down the coordinates of A .
10) Determine the equation of the line passing through A and perpendicular to 4x- 3y +12= 0.
11) ABCD is a rhombus. The coordinanates of A and C are (3,6) and (-1,2) respectively. Write down the equation of BD.
2) √3, 60°, 5/2
3) -4 4) 2/3 5) x - √3 y - 4√3=0 6) 2x - 7y -3=0 7) 3x - 2y +4=0 8) x + 2y -4=0 9) (-3,0) 3x + 4y +9=0 10) x + y =5
LONG ANSWER TYPE QUESTIONS
1) Find the equation of the line perpendicular to the line joining the points A(1,2) and B (6,7) and passing through the point which divides the line segment AB in the ratio 3:2.
2) A straight line passes through P(2,1) and cuts the x and y-axis at the point A, B respectively. If BP: PA= 3:1, find
a) the coordinates of A and B.
b) the equation of the line AB .
3) A straight line makes positive intercepts on the coordinanate axes whose sum is 7. If the line passes through the point (-3,8), find the equation.
4) if the coordinates of the vertex A of a square ABCD are (3,-2) and the equation of the diagonal BD is 3x - 7y+ 6= 0, find the equation of the diagonal AC. Also , find the coordinates of the centre of the square.
5) Find the equation of the perpendicular from the point (1,-2) on the line 4x- 3y= 5. Also, find the coordinanates of the foot of the perpendicular.
a) Find the coordinates of A and B.
b) Find the equation the line through P and perpendicular to AB.
7) Find the value of P if the lines, 5x- 3y+2= 0 andn6x - py +7= 0 are perpendicular to each other. Hence, find the equation the line passing through (-2,-1) and parallel to 6x- py+ 7= 0.
8) Points A and B have coordinanates (7,-3) and (1,9) respectively. Find
a) the slope of AB .
b) the equation of the perpendicular bisector of the line segment AB .
c) the value of P if (-2, p) lies on it.
9) A and B are two points on the x-axis and y-axis respectively. P(2,-3) is the mid point of AB . Find
a) the co-ordinate of A and B.
b) slope of line AB.
c) equation of line AB.
1) x+ y -9=0
2) (8/3,0),(0,4) 3x + 2y -8=0
3) 4x + 3y -12=0 4) 7x + 3y -15=0 , (3/2,3/2)
5) 3x + 4y +5 =0, (1/5,-7/5)
6) (6,0),(0,-3) 2x + y - 7=0
7) -10, 3x + 5y +11=0
8) -2 2y - x =2 9
9) (4,0),(0,6). 3/2 3x - 2y -12=0
SIMILARITY (AS A SIZE TRANSFORMATION)
1) Figures which have exactly the same shape , but not necessary the same___, are said to be similar.
a) angle b) side c) size d) volume
2) all regular polygons having the same number of ___are similar.
a) sides b) medians c) diagonals d) altitudes
3) Two circles are always :
a) congruent b) similar c) enarged d) concentric
4) in size transformation, the given figure is called an object and the resulting figure is called its:
a) pre- image b) image c) post image d) enlarge object
5) let K be the scale factor of a given size transformation . Then K< 1 as the transformation is a:
a) enlargement b) identify transformation c) transformation d) preserved
6) Each side of the resulting figure= ____ times the corresponding side of the given figure.
a) k² b) k c) k³ d) 2k
7) The transformation is an____ if k= 1 where k is the scale factor of a given size transformation.
a) identify transformation b) reduction c) enlargement d) none
8) in case of solids, we have volume of the resulting figure = ____x (volume of the given figure), where k is the scale factor.
a) k b) k² c) k³ d) 3k
9) if scale factor, k= 1/p, then area of the model= ____ x (area of the actual figure).
a) k² b) k c) k² d) 1/p
10) Let the map of a plane figure be drawn to the scale 1: p. Then scale factor k= ____, length in the map= k x (actual length (.
a) p/1 b) 1/p c) 1/k d) k
1c 2a 3b 4b 5c 6b 7a 8c 9a 10b
SHORT ANSWER TYPE OF QUESTIONS
1) ∆ ABC with sides AB= 12cm, BC= 8cm and AC= 14cm is enlarged to ∆A'B'C' such that the smallest side of ∆ A'B'C'= 12cm. Find the scale factor and use it to find the length of the other sides of the image A'B'C'. 3/2, 18cm, 21cm
2) ∆ ABC is reduced by scale factor 0.72. if the area of ∆ ABC is 62.5cm², find the area of the image. 32.4 cm²
3) A rectangle hving an area of 60cm² is transferred under enlargement about a point in space. If the area of its image is 135cm², find the scale factor of the enlargement. 1.5
4) In the map of a rectangular plot of land the length= 2.5 cm and breadth=1.4cm. if the scale 1: 1000, then find the area of the plot in m². 35000 cm²
5) The surface area of a solid is 5m², while surface area of its model is 20 cm². Find
a) the scale factor. 1/50
b) the volume of the solid if the volume of the model is 100cm³. 12.5 m³
6) Two bottles of sauce of circular cross-section are completely similar in every respect. One is 24cm high and the other is 32 cm high.
a) Calculate the external diameter of the smaller bottle, given that the corresponding diameter for the other bottle is 8cm. 6cm
b) The smaller bottle can hold it 270cm³ of sauce. How much souce can the bigger bottle hold? 640 cm³
7) The model of a building is constructed with scale factor 1:30.
a) If the height of the model is 80cm, find the actual height of the building in metre. 24
b) If the actual volume of the tank on the tap of the building is 27m³, find the volume of the tank on the top of the model . 1000 cm³
8) Two similar jugs have heights of 4cm and 6cm respectively. If the capacity of the smaller jug is 48 cm³, find the capacity of the larger jug. 162cm³
9) Two similar cylindrical tins have base radii of 6cm and 8cm respectively. Find the capacity of the smaller tin, if the capacity of the largest tin is 256 cm³. 108cm³
LONG ANSWER TYPE QUESTIONS
1) The model of a ship is made to a scale 1:200.
a) The length of the model is 4m. Calculate the length of a ship. 800m
b) The area of the deck of the ship is 160000m². Find the area of the deck of the model. 4m²
c) The volume of the model is 200 litres . Calculate the volume of the ship in m². 1600000
2) On a map drawn to a scale of 1:250000 a triangular plot of land has the following measurements: AB= 3cm, BC = 4cm, angle ABC= 90°
Calculate:
a) the actual length of AB in km. 7.5 km
b) the area of the plot in km². 37.5 km²
3) On a map drawn to a scale of 1:25000, a rectangular plot of land ABCD has the following measurements .
a) The diagonal distance of the plot in km. 5km
b) The area of the plot in km². 12 km²
4) The scale of a model ship is 1:300.
a) if the length of the model is 250cm, find the actual length in m. 750m
b) if the desk area of the model is 1 m², find the deck area of the ship and the cost of painting it at Rs10 per m². 90000 m², Rs900000
c) If the volume of the ship is 108000000 m³, find the volume of the model. 4m²
5) The dimension of the model of the multistoreyed building are 1m x 60 cm x 1.25m. if the model is drawn to a scale 1:60, find the actual dimensions of the model in metres . Also find
a) the floor area of a room of the building, whose area in the model 250 cm². 60m x 36m x75m , 90m²
b) the volume of the room in the model whose actual volume is 648 m³. 3600 cm³
SIMILARITY OF TRIANGLES
1) If in two triangles ABC and PQR, AB/QR = BC/PR = CA/PQ, then
a) ∆ PQR~ ∆ CAB b) ∆ PQR~∆ ABC c) ∆ CBA~ ∆ PQR d) ∆ BCA~ ∆ PQR
2) If in a triangles DEF and PQR, angle D= angle Q, and angle R = angle E, then which of the following is not true ?
a) EF/PR= DE/QR b) DE/PQ= EF/RP c) DE/QR = DF/PQ d) EF/RP= DE/QR
a) 4.5 b) 3 c) 3.6 d) 2.4
4) If ∆ ABC~∆ EDF and ∆ ABC is not similar to ∆ DEF, then which of the following is not true?
a) BC xEF= AC x FD
b) AB x EF = AC x DE
c) BC x DE = AB x EF
d) BC x DE = AB x FD
5) If ∆ ABC~ ∆ PQR, and angle P= 50° and angle B =60°, then angle R is
a) 80° b) 70° c) 60° d) 50°
6) In ∆ LMN and ∆ PQR, if Angle L= 50°, angle M =70°, angle P= 60° and angle Q= 70°, then
a) ∆ LMN~ ∆ RQP b) ∆ LMN ~∆ PQR c) ∆ LMN ~ ∆ PRQ d) ∆ LMN ~ ∆ RQP
a) 10cm b) 12cm c) 15 cm d) none
8) The perimeter of two similar triangles are 30cm and 20cm. If one side of first triangle is 12cm, then the corresponding side of the second triangle is
a) 10cm b) 8cm c) 7 cm d) 6cm
9) In ∆ ABC and ∆ DEF, angle B= angle E, angle F= angle C and AB= 3 DE, then the two triangles are
a) congruent but not similar
b) similar but not congruent
c) neither congruent not similar
d) congruent as well similar
a) 6 cm b) 7cm c) 4.8cm d) 5.2 cm
11) If ∆ ABC~ ∆ QRP, ar(∆ ABC)/ar(∆ PQR)= 9/4, AB = 18cm, BC= 15cm, then PR is equal to
a) 10cm b) 12 cm c) 20/3 cm d) 8 cm
12) if it is given that ∆ ABC~∆ PQR with BC/QR= 1/4, then ar(∆ PQR)/ar(∆ ABC) is equals to
a) 4 b) 16 c) 1/4 d) 1/16
13) ∆ ABC~∆ DEF such that AB= 9.1cm and DE=6.5 cm. if perimeter of ∆ DEF is 25cm, then the perimeter of ∆ ABC is
a) 35cm b) 28 cm c) 42cm d) 40cm
14) ∆ AOQ~∆ BOP, AO=10cm, BO= 6cm and PB=9cm, then length of AQ is:
a) 10cm b) 11cm c) 12cm d) 15 cm
15) If P, Q, R are respectively the midpoints of the sides BC, CA and AB of ∆ ABC, then the ratio of the areas of ∆ PQR to ∆ ABC is
a) 1:2 b) 2:1 c) 1:4 d) 4:1
16) If in ∆ ABC and ∆ DEF AB/DE= BC/FD, they will be similar when
a) angle B= angle E
b) angle A= angle D
c) angle B= angle D
d) angle F= angle F
a) ∆ PQR b) ∆ RPQ c) ∆ QPR d) angle PRQ
a) 3cm b) 3.5 cm c) 4 cm d) 4.2cm
19) In the figure, if D, E and F are made points of the sides BC, AC and AB respectively. then the two triangles ABC and DEF are :
a) similar b) congruent c) both a and b d) neither similar nor congruent
20) In the given figure, LM || NQ and LN || PQ. If MP= (1/3) MN, then the ratio of the areas of ∆ LMN and ∆ QNP is
a) 9:4 b) 16 : 4 c) 25 :16 d) 64:9
21) M and N are respectively the points on the sides XY and XZ of a triangle XYZ such that XM= 3cm, MY = 5 cm, and YZ =12cm. If MN || YZ, then the length of MN is
a) 6cm b) 5cm c) 4.5cm d) 4 cm
a) 3cm b) 3.2 cm c) 3.8cm d) 4.5 cm
a) 10cm b) 8cm c) 7cm d) 6cm
24) The perimeters of two similar triangles are ABC and XYZ are 60 cm and 48cm respectively. If XY =8cm, then the length of AB is
a) 12 cm b) 11cm c) 10 cm d) 9cm
a) 25:81 b) 16:9 c) 9: 16 d) 64: 25
1a 2b 3c 4c 5b 6a 7a 8b 9b 10c 11a 12b 13a 14d 15c 16c 17c 18a 19a 20d 21c 22d 23d 24c 25a
Short Answer Type Questions
a) Prove ∆ TPS ~ ∆ TRQ
b) Find SP if TP= 18cm, RQ = 4cm and TR= 6 cm. 12cm
a) prove ∆ ADB ~∆CDA.
b) if BD = 18cm, CD= 8cm, find AD . 12cm
3) in the given figure ∆ ABC and ∆ AMP are right angled at B and M respectively. Given AC= 10 cm, AP=15 cm and PM= 12cm
a) Prove ∆ ABC~∆ AMP
b) find AB and BC . 6cm, 8cm
a) Prove that ∆ ACD~∆ BCA
b) find BC and CD. 6.4cm, 2.5cm
c) find ar(∆ ACD: ar(∆ ABC ). 25:64
5) ABC is a right angled triangle with angle ABC=90°. D is any point on AB and DE is perpendicular to AC, prove that :
a) ∆ ADE~∆ACB
b) If AC= 13cm, BC= 5cm and AE= 4cm, find DE and AD. 1.66cm, 4.33 cm
Long answer type questions
1) In the figure, ABCD is a trapezium in which AB|| DC and the diagonals AC and BD Intersect at O. Show that
a) ∆ OCD~∆OAB,
b) If OA= (2x +1) cm, OB= (5x -3)cm, OC= (6x -5) cm and OD=(3x -1) cm, find the value of the x. 4/3
a) Show that ∆ ADE~∆ABC
b) Find AD/AB and DE/BC . 4/7
c) prove that ar(∆ DEF): ar(∆DEC)= 4:11. 4/7
4) Through the midpoint M of the side CD of a parallelogram ABCD, the line BM is drawn intersecting AC at L and AD produced at E. Show that EL= 2BL.
5) In a trapezium ABCD, AB= (1/2) CD, EF drawn parallel to AB cuts AD at F and BC at E, such that BE/EC = 3/4. If diagonal BD Intersect EF at G, find EF/AB. 10/7
LOCI
Choose the correct option:
1) The locus is the ____traced out by a moving point which moves according to some given geometrical conditions.
a) line b) circle c) path d) arc
2) The plural of locus is:
a) locci b) loci c) Locus d) Losai
3) The locus of a point equidistant from two___ lines is the bisector of the angles between the lines.
a) intersecting b) perpendicular c) parallel d) same
4) The locus of a point equidistant from two given points is the of the___of the line segment joining the points.
a) perpendicular b) bisector c) perpendicular bisector d) parallel
5) The Locus of a point, which is equidistant from two parallel straight lines, is straight line___ to the given lines and midway between them .
a) perpendicular b) parallel c) bisects d) none of these
6) The locus of the centre of a wheel, which moves on a straight horizontal road, is a straight line parallel to the road and at a distance equal to the___ of the wheel.
a) diameter b) circumference c) radius d) same
7) The Locus of a point, which is made inside a circle and is equidistant from two points on the circle, is the diameter of the circle which is____ to the chord of the circle joining the given points.
a) perpendicular b) parallel c) bisected d) none
8) The locus of the____ of all parallel chords of a circle is the diameter of the circle which is perpendicular to the given parallel chords.
a) end points b) midpoint points c) points on the circumference d) none
9) The locus of a point which is equidistance from the two given concentric circles of radii r₁ and r₂ is the circle of radius___ concentric with the given circles. It lies midway between them.
a) (r₁ +r₂) b) (r₁ +r₂)/2 c) (r₁ -r₂)/2 d) (r₁ -r₂)
10) If a fixed points, then the locus of a point P is such that angle APB=90° is the circle with AB as:
a) diameter b) radius c) arc d) none
1c 2b 3a 4c 5b 6c 7c 8b 9b 10a
Short Answer Type Questions
1) State the locus of a point in a rhombus ABCD which is equidistant :
i) From AB and CD
b) From the vertices A and C.
2) Construct a quadrilateral ABCD in which AB=5cm, BC= 4cm, angle B=60°, AD= 5.5cm and D is equidistant from AB and BC.
3) Construct the Locus of points inside the triangle ABC, which are equidistant from B and C. Mark the point P which is equidistant from AB, BC and also equiistant from B and C.
4) Construct angle ABC=120°, where AB= BC= 5cm. Mark two points D, E which satisfy both the following conditions :
a) equidistant from BA and BC
b) at a distance of 5cm from B.
Point E is on the side of reflex angle ABC. Join AE, EC, CD and AD. Describe the figures
i) AECD
ii) ABD
iii) ABE.
5) Describe completely the locus of points in each of the following cases :
i) midpoint of radii of a circle.
ii) Center of a ball, rolling along a straight line on a level floor.
iii) point in a plane equidistant from a given line.
6) Draw two intersecting lines to include an angle of 30°. Use ruler and compass to locate points which are equidistant from these lines and also 2cm away from their point of intersection. How many such points exists ?
7) Draw a line segment AB=3cm. Draw the locus of a point P which moves at a distance of 5 cm from AB.
1) AC BD
4) kite equilateral triangle isosceles triangle
5) A concentric circle with half the radius
A parallel straight line at a height of half the radius of the ball.
A pair of parallel lines parallel to the given lines in the plane.
6) 4 points
Long Answer Type Questions
1) Draw a line segment AB= 8cm. Mark C, the midpoint of AB. Draw and describe the locus of a point which is
a) 2cm from AB
b) 4cm from C.
Mark the points E, F, G, H which satisfy both the above conditions .
i) Describe the figure EFGH.
ii) What kind of triangle is ECF ?
2) Construct a right angled triangle PQR such that PQ= 6cm, QR= 3.5cm and angle PQR= 90°. Bisect Ang P internally and mark a point Z on this bisector such that PZ= 4cm. Find the points which are 4cm from Z and also 4cm from the line QR.
3) Construct an isosceles triangle ABC such that AB= 6cm, BC= AC= 4cm. Bisect angle C internally and mark a point P on this bisector such that CP= 5cm. Find the points which are 5cm from P and also 5cm from the line AB.
4) Construct triangle ABC in which AB= 6cm, BC= 7cm and angle ABC=60°. Locate by construction the point P such that
a) P is equidistant from B,C.
b) P is equidistant from BC and AC.
c) Measure and record the length of PB.
5) Using ruler compass construct.
i) a Triangle ABC in which AB =5.5cm, BC =3.4cm and CA= 4.9cm.
ii) the Locus of points equidistant from A and C.
iii) a circle touching AB at A and passing through C.
3) rectangle
Equilateral triangle
4) 4cm
ANGLE AND CYCLIC PROPERTIES OF A CIRCLE
Multiple Choice Questions
a) 20 b) 40 c) 60 d) 10
2) In the figure, angle PQR=100°, where P, Q and R are points on a circle with centre O, then angle OPE=
a) 15 b) 12 c) 10 d) 8
a) 60 b) 70 c) 80 d) 100
a) 30 b) 60 c) 90 d) 45
a) 50 b) 40 c) 60 d) 70
6) In the figure A, B and C are 4 points on a circle. AC and BD interesect at a point E such that angle BEC = 130° and angle ECD= 20, then angle BAC=
a) 100 b) 102 c) 50 d) 110
7) ABCD is a cyclic quadrilateral whose diagonals Intersect at a point E. If Angle DBC=70°, and angle BAC=30°, then angle BCD=
a) 60 b) 70 c) 80 d) 75
a) 50 b) 45 c) 40 d) 35
a) 18 b) 28 c) 32 d) 46
10) ABCD is a parallelogram. A circle passes through A and D, cuts AB at P and DC at Q. If ang BPQ=80°, then angle ABC is
a) 60 b) 75 c) 80 d) 105
11) In the given figure, AB and CD are two equal chords of a circle with centre O. OP and OQ are perpendicular on chord AB and CD respectively. If Angle POQ= 140°, then angle APQ is
a) 70 b) 80 c) 95 d) 105
12) In the given figure, O is the centre of the circle. If Angle OPR and OQR are 40° and 30° respectively then, the measure of angle POQ is
a) 160 b) 150 c) 140 d) 130
a) 40 b) 50 c) 60 d) 75
a) 115 b) 120 c) 135 d) 150
15) In the given figure, O is the centre of the circle. If Ang PAQ=120° and angle RQS=25°, then the measure of angle PRQ is
a) 95 b) 105 c) 115 d) 135
a) 60 b) 50 c) 70 d) 80
17) ABCD is a cyclic quadrilateral such that AB is a diameter of the circle circumscribing it and angle ADC=130°, then angle BAC is
a) 80 b) 50 c) 40 d) 30
a) 30 b) 45 c) 90 d) 60
19) In the figure, O is the centre of the circle. The angle subtended by the arc BCD at the centre is 140°, BC is produced to P, then angle BAD+ angle BCD=
a) 160 b) 170 c) 180 d) 210
20) In the figure, chord ED is parallel to the diameter AC of the circle. Given angle CBE=65°, then angle DEC=
a) 35 b) 30 c) 25 d) 20
a) angle A B) Ang B c) angle C d) none
a) 86 b) 84 c) 82 d) 80
1b 2c 3c 4d 5a 6d 7c 8d 9b 10c 11a 12c 13b 14a 15a 16c 17c 18d 19c 20c 21a 22c
Short Questions
1) In the given figure, angles subtended by arc AC and BC at the centre O of the circle are 55° and 155° respectively. Find angle ACB. 75
2) BC is a chord of a circle with centre O. A is a point on major arc BC as shown in the figure. Show that angle BAC+ angle OBC= 90°.
3) Two circles Intersect at two points A and B. AD and AC are diameters of the two circles. Prove that B lies on the line segment DC.
4) ABCD is a cyclic quadrilateral whose diagonals Intersect at a point E, if Ang DBC=70°, angle BAC= 30°, find angle BCD. Further, if AB= BC, find angle ECD. 80,50
5) In the given figure, AB is a diameter of the circle. Angle BDC=20° and angle CBD=25°. Find angles AED and ACD. 65,45
6) In the given figure, ABCD is a cyclic quadrilateral O is the centre of the circle. If Angle COD=120° and angle BAC= 30°, find angles BOC and BCD. 60,90
a) angle BCD. 115
b) angle ADB. Hence, show that AC is a diameter of the circle. 45
8) In the figure A, D, B, C are four points on the circumference of a circle with centre O. arc AB= 2 arc BC and angle AOB= 108°. Calculate in degree
a) Ang ACB
b) angles CAB
c) Ang ADB . 54,27,126
9) In the given figure, AB is a diameter of the circle. PQ is a chord such that angle BAPA= Ang ABQ. Show that ABQP is a cyclic trapezium.
Long Answer Type Questions
2) In the given figure, AC is the diameter of the circle with Centre O. CD and BE are parallel, angle AOB=80° and angle ACE= 10°, calculate the angles BEC, BCD, CED. 50,100,30
3) In the figure, O is the centre of the circle. Angle AOE= 150°, angle DAO= 51°. Calculate the measure of angles BEC, EBC. 51,105
4) Two circles Intersect at P and Q. Through P, a straight line APB is drawn to meet the circles in A and B. Through Q, a straight line is drawn to meet the circles at C and D. Show that AC is parallel to BD.
TANGENT PROPERTIES OF CIRCLES
Multiple Choice Questions
1) In the given figure, TAS is a tangent to the circle, with centre O, at point A. If Angle OBA=32°, then the value of x will be:
a) 19 b) 38 c) 58 c) 76
2) A point P is 10cm from the centre O of a circle. The length of the tangent drawn from P to the circle is 8cm. The radius of the circle is equal to:
a) 4cm b) 5cm c) 6cm d) 8cm
3) In the given figure, PT is a tangent to the circle with centre O. If OT= 6cm and OP= 10cm, then the length of tangent PT is
a) 8cm b) 12cm c) 10cm d) 16cm
4) In the given figure, AC is a chord of the circle and AOC is its diameter such that angle ACB=50°. If AT is the tangent to the circle at a point A, then angle BAT is equal to
a) 65 b) 60 c) 50 d) 40
5) In the figure, AT is a tangent to the circle with centre O such that OT= 4cm and angle OTA=30°. The length of AT is
a) 4cm b) 2cm c) 2√3cm d) 4√3 cm
6) In the figure, chords AB and CD intersect at P. If PA= 6cm, PB= 5cm and CD= 13cm, then the length of PC is
a) 10cm b) 8cm c) 6cm d) 5cm
7) In the figure, PA and PB are tangents to the circle with centre O such that angle APB=50°, then the measure of angle OAB is
a) 37 b) 36 c) 50 d) 25
8) Tangents PQ and PR are drawn from an external point P to a circle with centre O, such that angle RPQ=30°. A chord RS is drawn parallel to the tangent PQR. Then angle RQS is
a) 40 b) 30 c) 25 d) 20
9) In the figure, PQ is a chord of a circle with centre O and PT is a tangent. If QPT = 60°, then angle PRQ is
a) 130 b) 120 c) 110 d) 100
10) In the figure, O is the centre of the circle. PT and PQ are tangents to the circle from an external point P. If Angle TPQ=70°, then angle TRQ is
a) 60 b) 58.5 c) 55 d) 50
11) In the figure, PA and PB are tangents to a circle with centre O, such that AP= 5cm and angle APB=60°, then the length of chord AB is
a) 9cm b) 5cm c) 4.5cm d) 4cm
12) In the figure, PQ is a tangent at a point C to a circle with centre O. If AB is a diameter and angle CAB=30°, then angle PCA is
a) 60 b) 58 c) 57 d) 56
13) In the figure, O is the centre of the circle, AB is a chord and AT is the tangent at a point A. If Angle AOB= 100°, then angle BAT is
a) 30 b) 40 c) 50 d) 100
14) Point P is 25cm away from the centre O of a circle and the length PT of the tangent drawn from P to the circle is 24cm. The radius of the circle is
a) 7cm b) 9cm c) 10cm d) 12 cm
15) In the figure, TP and TQ are two tangents to a circle with centre O such that, angle POQ=110°, the value of angle PTQ is
a) 60 b) 65 c) 70 d) 75
16) In the figure, AB is the diameter of a circle with centre O and AT is a tangent. If Angle AOQ= 60° , then angle ATQ is
a) 60 b) 50.5 c) 49 d) 30
17) In the figure, AOB is a diameter of a circle with centre O and AC is a tangent to the circle at Q. If Angle BOC=130°, then angle ACO is
a) 35 b) 38 c) 120 d) 40
18) In the figure, CP and CQ are tangents to a circle with centre O. ARB is another tangent touching the circle at R. If CP= 11cm and BC= 7 cm, then the length of BR is
a) 3.5cm b) 4cm c) 3cm d) 11cm
19) In the figure, PA and PB are two tangents from an external point P to a circle with centre O. If Angle PBA= 65°, then angle OAB is
a) 15 b) 25 c) 35 d) 45
20) In the figure, PA and PB are tangents to the circle with centre O. If Angle APB= 60°, then angle OAB is
a) 40 b) 30 c) 25 d) 20
1c 2c 3a 4c 5c 6a 7d 8b 9b 10c 11b 12a 13c 14c 15c 16a 17d 18b 19b 20b
Short Answer Type Questions
1) In the figure PA and PB are tangents to the circle drawn from an external point P. CD is another tangent touching the circle at Q. If PA= PB= 12cm and QD= 3cm, find the length of PD. 9cm
2) In the figure, PA and PB is a pair of tangents drawn to a circle having its centre at O. If Angle APB= 52°, find angles PAB, PBA. 64,64
3) A point P is 15cm from the centre of a circle. The radius of the circle is 5cm. Find the length of the tangent drawn to the circle from the point P. 10√2
4) In the figure, the circle touches the sides BC, CA and AB of ∆ ABC at D, E and F respectively. If AB= AC, show that BD= CD.
5) A circle is touching the side BC of a ∆ ABC at P and touching AB and AC produced at Q and R respectively. Show that AQ= (1/2) perimeter of ∆ ABC.
6) Show that the tangents drawn at ends of a diameter of a circle are parallel.
7) A quadrilateral ABCD is drawn to circumscribe a circle, as shown in the figure. Show that AB+ CD= AD+ BC.
8) In the figure, a circle touches all the four sides of a quadrilateral ABCD with AB= 6cm, BC=7cm and CD= 4cm. What is the length of AD? 3cm
9) In the figure, O is the centre of the circle, PQ is a tangent to the circle at A. If angle PAB =58°, find angles ABQ and AQB. 32,26
Long Answer Type Questions
1) PQ is a chord of length 8cm of a circle of radius 5cm. The tangents at P and Q intersect at a point T. Find the length of TP. 20/3 cm
2) In the figure, AB is diameter of a circle with centre O and QC is a tangent to the circle at C. If Angle CAB=30°, find angles CQA, CBA. 30,60
3) ABC is a right angle triangle, right angled at B. A circle is inscribed in it. The length of the two sides containing the right angle are 6cm and 8cm. Find the radius of the circle. 2cm
4) Two tangents PA and PB are drawn to the circle with centre O, such that angle APB= 120°. Show that OP= 2AP.
5) Two tangents TP and TQ are drawn to a circle with centre O from an external point T. Show that angle PTQ= 2 angle OPQ.
6) A circle is inscribed in a ∆ ABC having sides 8cm, 10cm and 12cm as shown in the figure. Find AD, BE, and CF. 7, 5, 3cm
CONSTRUCTION
Short Answer Type Questions
1) Draw a circle with centre O and radius 3.1cm. Take a point P on it. Draw a tangent to the circle at point P.
2) Draw a circle of radius 2.5cm and take a point T on it. Draw a tangent to the circle at a point T, without using the centre.
3) Draw a circle with centre O and radius 2.9cm. Take a point P outside the circle at a distance of 4.6cm from its centre.
Draw two tangents to the circle from the point P.
4) Draw a circle of radius 4cm. Draw any diameter of the circle. At the end points of the diameter of the circle, draw tangents to the circle. Are they parallel?
5) Draw a circle of radius 4.1cm. Draw two tangents to the circle which are inclined to each other at an angle of 60°.
6) Draw two concentric circles of radii 2.5cm and 4cm. Draw a tangent to the inner circle from a point on the outer circle.
Long Answer Type Questions
1) Draw a line segment AB of length 8cm. Taking A centre, draw a circle of radius 4 cm and taking B as Centre, draw another circle of radius 3cm. Construct tangents to each circle from the centre of the other circle.
2) Draw a circle of radius 6 cm. Take a point P, at a distance of 8cm from its centre. From P, draw two tangents to the circle, which are inclined to each other at an angle of 45°. Measure the length of its tangent.
3) Using ruler and compass construct a ∆ ABC, such that AB =2.6cm, BC =4.1cm and AC= 5.1cm. Draw the circumscribed circle of ∆ ABC.
4) Draw an isosceles triangle PQR , in which PQ= QR= 5 cm and PR= 3.5cm. Draw the incircle of the triangle.
5) Using a ruler and a pair of compasses only, construct, a triangle ABC, given AB= 4cm, BC= 6cm and angle ABC= 90°. Then construct a circle passing through the points, A, B, C and mark its centre as O.
6) Construct a regular hexagon of side 4 cm. Construct a circles circumscribing the hexagon.
7) Draw a regular hexagon of side 2.2cm. Inscribe a circle in it.
VOLUME AND SURFACE AREA OF SOLIDS
1) If the volume of a right circular cone of height 9cm is 48π cm³, then diameter of its base is
a) 6cm b) 7cm c) 8 cm d) 10 cm
2) A hemispherical bowl has a radius of 3.5cm. The volume of water it would contain would be :
a) 86.5cm² b) 89.8cm³ c) 92.5cm⅔ d) 93.5cm³
3) The height of a right circular cone is 12cm. If its volume be 100π cm³, then its slant height is:
a) 12cm b) 13cm c) 15cm d) 16cm
4) A conical flask of base radius r and height h is full of milk. The milk is now poured into a cylindrical flask of radius 2r. What is the height to which the milk will rise in the flask?
a) h/2 b) h/6 c) h/9 c) h/12
5) the radius of base and the height of a right circular cylinder are each increased by 20%. The volume of the cylinder will increase by
a) 72.8% b) 80% c) 82% d) 92%
6) A sphere of radius R has volume equal to that of a cone of radius R, the height of the cone is:
a) R b) 3R c) 3R d) 4R
7) A sphere and a cube have the same surface area. The ratio of their volume is:
a) √6: √π b) √3: √π c) π: √2 d) √5:π
8) A medicine capsule is in the shape of a cylinder of diameter 0.5cm, with two hemispheres stuck to each of its ends. The length of entire capsule is 2cm. The capacity of the capsule is
a) 0.21cm³ b) 0.42cm³ c) 1.33cm³ d) 0.33 cm³
9) If two solid hemispheres of same base radius r are joined together along their bases, then curved surface area of this new solid is:
a) 4πr² b) 6πr² c) 3πr² d) 8πr²
10) A right circular cylinder of radius r cm and the height h cm (h > 2r) just encloses a spher of a diameter:
a) r cm b) 2r cm c) h cm d) 2h cm
11) During conversion of solid from one shape to another, the volume of the new shape will be
a) increase b) decrease c) remains unaltered d) be doubled
12) Volume up two Sphere are in the ratio 64 : 27. The ratio of their surface area is:
a) 3:4 b) 4:3 c) 9 : 16 d) 16:9
13) A solid metallic sphere of diameter 21cm is melted and recast into a number of smaller cones, each of diameter 7cm and height 3cm. The number of cones so firmed is:
a) 121 b) 123 c) 125 d) 126
14) A hemispherical bowl of internal diameter 30cm contains some liquid. This liquid is to be filled into cylindrical shaped bottles, each of diameter 5 cm and height 6cm. The number of bottles necessary to empty the bowl is:
a) 30 b) 40 c) 55 d) 60
15) The base radius and height of a right circular solid cone are 2cm and 8cm respectively. It is melted and recast into sphere of diameter 2cm each. The number of spheres so formed is:
a) 6 b) 8 c) 10 d) 12
16) In a cylinder, if radius is halved and height is doubled, the volume will be
a) same b) doubled c) halved d) four times
17) The radius of a sphere is 3r, then its volume will be:
a) 4πr³/3 b) 36πr³ c) 8πr³/3 d) 32πr³/3
18) A cone is 8.4 cm high and the radius of its base is 2.1cm. it is melted and recast into a sphere. The radius of the sphere is:
a) 4.2 b) 2.1cm c) 2.4cm d) 1.6 cm
19) In a cylinder, radius is doubled and height is halved, curved surface area will be:
a) halved b) doubled c) same d) four times
20) The total surface area of a cone whose radius is r/2 and slant height 2L is
a) 2πr(L+ r) b) πr(L+ r/4) c) πr(L+ r) d) 2πrL
21) The radius of two cylinders are in the ratio of 2:3 and their heights are in the ratio 5:3. The ratio of their volumes is:
a) 10:17 b) 20:27 c) 17:27 d) 20:37
22) The circumference of the base of a cylindrical vessel is 132cm and its height is 50 cm. How many litres of water can it hold?
a) 34.39 litres b) 78.50 litres c) 69.30 litres d) 67.67 litres
23) The diameter of a cone is 28cm and slant height is 10.5cm. Then, its lateral surface area is
a) 480cm² b) 490cm² c) 462cm² d) 466cm²
24) The diameter of a cone is 10cm and vertical height is 12cm. Then, the area of curved surface area is:
a) 205.15 cm² b) 214.16 cm² c) 204.28 cm² d) 241.20 cm²
25) The lateral surface area of a cone is 60π cm². If the slant height of the cone be 8cm, then the diameter of its base is
a) 25 cm b) 18cm c) 12cm d) 15 cm
1c 2b 3b 4d 5a 6d 7a 8d 9a 10b 11c 12d 13d 14d 15b 16c 17b 18b 19c 20b 21b 22c 23c 24c 25d
SHORT ANSWER TYPE QUESTIONS
1) 2.2 dm³ of copper is to be drawn into a cylindrical wire of diameter 0.50cm. find the length of the wire.
2) A wall of diameter 1m is dug 7m deep. The earth taken out of it is completely used for making a conical body of scare-crow. The base diameter of the body is 2m. Find the height of scare crow.
3) The total surface area of a right circular cone of slant height 13cm is 90π cm². Calculate
a) its radius in cm.
b) Its volume in cm³, in terms of π.
4) If the volume of two cylinders are in the ratio 128:75 then, find the ratio of their curved surface areas. It is given that the ratio of their heights is 2:3.
5) How many metres of cloth 5m wide will be required to make a conical tent, the radius of whose base is 7m and whose heights is 24m ?
6) How long would it take to fill a conical vessel whose diameter of base is 20cm and depth is 21cm, if it is filling through a pipe of diameter 5mm, at the rate of 10 m/minutes.
7) An iron sphere of radius 5cm has been melted and recast into smaller sphere each of radius 2.5cm. How many smaller spheres are made.
8) A cone and a hemisphere have equal bases and equal volume. Find the ratio of their heights.
1) 112m 2) 5.25m 3) 5cm, 100π cm³ 4) 16:15 5) 110m 6) 11.20 min 7) 8 8) 2:1
LONG ANSWER TYPE QUESTIONS
1) The given solid figure is a cylinder surmaunted by a cone. The diameter of the base of the cylinder is 6cm. The height of the cone is 4cm and the total height of the solid is 26cm. (π= 22/7). Find
a) volume of the solid
b) curved surface area of the solid
Give your answers correct to the nearest whole number.
2) A solid metallic sphere of radius 6cm is melted and made into a solid cylinder of height 32cm. Find the
a) radius of the cylinder.
b) curved surface area of the cylinder (π= 3.1).
3) The volume of a conical tent is 1232 m³ and the area of the base floor is 154 m². Find
a) radius of the floor.
b) height of the tent
c) length of the canvas required to cover this conical tent, if its width is 2m.
4) The given figure represents a hemisphere surmounted by a conical block of wood. The diameter of their bases is 6cm each and the slant height of the cone is 5cm. Find
a) the height of the cone
b) the volume of the solid.
5) A plumber fits a pipe of internal radius 10cm from a tap to a cylindrical tank, which is 5m in radius and 2m deep. If the water flows through the pipe at the rate of 3 km/hr, in how much time will tank filled?
6) Two solid iron poles are lying one over other. The pole at the lower position has height 220 cm and base diameter 24cm, whereas the pole above it has height of 60cm, and base diameter 16cm. Calculate the weight of the pole, if 1 cm³ of iron weight 10g.
7) A small cone is cut off at the top of a cone by a plane parallel to the base. The volume of the small cone is 1/8 of the volume of the bigger cone. At what height above the base is the section made, it is given that height of the complete cone is 40 cm ?
8) From a solid cylinder whose height is 2.4 cm, and diameter 1.4cm, a conical cavity of the same height and same diameter is hollowed out. Find the total surface area of the remaining solid to the nearest cm².
1) 632cm³, 443 cm² 2) 3cm, 595.2 cm² 3) 7m, 24 m, 275m 4) 4cm, 94.29 cm³ 5) 100 min 6) 1115.328 kg 7) 20cm 8) 18 cm²
TRIGONOMETRIC IDENTITIES
1) {(1+ tanθ)/(1+ tanθ)}² =
a) 1 b) tan²θ c) tanθ d) 4
2) (sinA - 2 sin³A)/(2cos³A - cosA) is equal to
a) secA b) tanA c) cotA d) 1
3) If tanx = cotx, then
a) x - = 90 b) x= y = 90 c) x + y= 90 d) y - x =90
4) (sec²θ -1)(1- cosec²θ) is equal to
a) -1 b) cotθ c) 0 d) cosecθ
5) √{(secθ -1)/(secθ +1)} + √{(secθ +1)/(secθ -1)} is equal to
a) 2cosecθ b) 2secθ c) 2tanθ d) 2sinθ
6) If sinθ + cosθ = a and secθ + cocosθ =b, then the value of b(a²-1) is
a) 2a b) a+ b c) 2b d) a - b
7) If sinθ + cosθ = √2cosθ, then the value of cosθ - sinθ is
a) √cosθ b) sinθ c) √sinθ d) cosθ
8) cosec²θ/(1 + cot²θ) =
a) 0 b) cosec θ c) 1 d) cotθ
9) sinθ + 2cosθ = 1, then the value of 2sinθ - cosθ =
a) 1 b) 2 c) √2 d) 0
10) If (sinθ + cosθ)(tanθ + cotθ) =
a) secθ + tanθ b) secθ c) secθ + coseθ d) cosecθ
11) sinθ/(1+ cosθ) + (1+ cosθ)/sinθ =
a) 2sinθ b) 2cosθ c) 2tanθ d) 2cosecθ
12) cosθ/(1- sinθ) + cosθ/(1+ sinθ) =
a) 2sinθ b) 2cosθ c) 2 cosecθ d) 2secθ
13) 5 tan²θ - 5 se²θ =
a) 5 b) -5 c) 1/5 d) 0
14) If secθ + tanθ =p, then (p²-1)/(p²+1)=
a) tanθ b) cosθ c) sinθ d) cocosθ
15) sin⁶θ + cos⁶θ + 3sin²θ. cos²θ =
a) 0 b) 1 c) 2 d) -2
16) If sinθ + cosθ = √3, then tanθ + cotθ =
a) 1 b) -1 c) 2 d) -2
17) secθ(1- sinθ)(secθ + tanθ) =
a) 0 b) 1/2 c) 1 d) none
18) 9 sec²θ - 9 tan²θ =
a) 1 b) 9 c) 8 d) 0
19) (1+ tanθ + secθ)(1+ cotθ - cosecθ =
a) 0 b) 1 c) 2 d) -1
20) (1+ tan²θ)/(1+ + cot²θ) =
a) sec²θ b) -1 c) cot²θ d) tan²θ
21) (secθ + tanθ)(1- sinθ =
a) secθ b) sinθ c) cocosθ d) cosθ
22) 2 cos²θ + 2/(1+ cot²θ) =
a) 1 b) 2 c) 0 d) 1/2
23) Simplified form of (3- tanθ)/(3cosecθ - secθ) is
a) cosθ b) sinθ c) cocosθ d) tanθ
1b 2b 3c 4a 5a 6a 7c 8c 9b 10c 11d 12d 13b 14c 15b 16a 17c 18b 19c 20d 21d 22b 23b
SHORT ANSWER TYPE QUESTIONS
Prove the following:
1) secθ/(secθ -1) + secθ/(secθ + 1) = 2cosec²θ.
2) √(sec²θ + cosec²θ) = tanθ + cotθ
3) (cosecθ - sinθ)(secθ - cosθ)(tanθ + cotθ) = 1
4) sinθ/(1+ + cotθ) - cosθ/(1+ tanθ) = sinθ - cosθ
5) sin⁴θ - cos⁴θ = 1- 2cos²θ
6) (1- sinθ)/(1+ sinθ) = (secθ - tanθ)²
7) (sin²x cos²y - cos²x sin²y) = sin²x sin²y.
8) Simplify: (sec²θ - 2 tan²θ)(1- sinθ)(1+ sinθ).
9) For what value of x, 2sin²x - cos²x = 2 ?
10) Find the value: tan²x + cot²x - sec²x cosec²x.
11) sinθ/(cotθ + cosecθ) - sinθ/(cotθ - cosecθ).
8) 1- 2 sin²θ 9) 90° 10) -2 11) 2
LONG ANSWER TYPE QUESTIONS
1) If tanθ + sinθ =m and tanθ - sinθ =n, show that m²- n²= 4 √(mn).
2) Show that: (secθ + tanθ -1)/(tanθ - secθ +1) =(1+ sinθ)/cosθ.
3) If tanθ + secθ = m, then find the value of (m²+1)/2m.
4) Express, a cosθ - b sinθ in terms of a, b and c, where a sinθ + b cosθ = c.
5) Show that: tanθ/(1- cotθ) + cotθ/(1 - tanθ) = tanθ + cotθ+ 1.
6) Show that: (secθ + tanθ -1)(secθ - tanθ +1)/tanθ = 2.
7) If x= a secθ + b tanθ and y= a tanθ + b secθ, then evaluate (a²- b²)/(x²- y²).
8) If x= a secm cos n, y= b secm sin n and z= c tan m, then evaluate x²/a² + y²/b² - z²/c².
9) Show that: 1/(secθ - tanθ) - 1/cosθ - 1/(secθ + tanθ).
10) Show that (1+ cotθ - cosecθ)(1+ tanθ + secθ) = 2.
3) secθ 4) ± √(a²+ b² - c²)
HEIGHT AND DISTANCES
1) If the length of the shadow of a pole is equal to its height, then the angle of elevation of Sun is
a) 30° b) 45° c) 60° d) 90°
2) If the length of the shadow of a tower is increasing, then angle of elevation of the Sun is
a) increasing b) first increasing, then decreasing c) decreasing d) not changed
3) if the height of a tower and the distance of the point of observation from its foot both are increased by 10%, then the angle of elevation of its top:
a) gets doubled b) remains unchanged c) gets tripled d) becomes half
4) If the angle of elevation of the sun is 60° and the length of the shadow of a tower is 30m, then the height of tower is
a) 3√3 b) √3 c) 30√3 d) 2√3
5) The angles of depression of two objects from the top of a 100m hill lying to its east are found to be 45° and 30°. The distance between the two objects is (√3=1.73)
a) 73.2m b) 107.5m c) 150 m d) 200 m
6) A kite is attached to a string. The length of the string, when the height of the kite is 60m and the string makes an angle 30° with the ground is
a)120m b) 30 m c) 50m d) 60m
7) The angle of elevation a plane 2x metres above the ground from a point x metres above the ground is θ. At this moment the angle of depression of a point just below the plane will be
a) sin(45-θ) b) cot(45-θ) c) 2θ d) θ
8) If the angles of elevation of the top of a vertical tower from two points A and B on the ground respectively 30° and 60°, then the ratio of the distances of A and B from the upper end of the tower is
a) √3 :1 b) 1:√3 c) √3+1:1 d) 1: √3-1
9) A ladder 15m long just reaches the top of a vertical wall. if the ladder makes an angle of 60° with the wall, then the height of the wall is
a) 6.5m b) 7.5m c) 8.5m d) 15/√3 m
10) A Tower stands vertically on the ground. From a point on the ground, which is 15m away from the foot of the tower, the angle of elevation of the top of the tower is found to be 60°, then height of the Tower is
a) 6√3m b) 15√3m c) 18m d) 25/√3m
11) A bridge, in the shape of a straight path, across a river, makes an angle of 60° with the width of the river. If the length of the bridge is 100 metres, then the width of the river is :
a) 50m b) 175.5m c) 92m d) 100m
12) An observer 1.5 m tall is 18.5m away from the tower. if the angle of the elevation of the top of the tower from his eyes is 45°, the height of the tower is
a) 15m b) 20m c) 8.5m d) 25.6m
13) The shadow of a tower, standing on a level ground, is found to be 40 m longer when the sun's attitude is 30° than when it was 60°. Then height of the tower is
a) 20m b) 10√3m c) 10m d) 20√3m
14) From a point on a bridge across a. river, the angles of depression of the banks on opposite sides of the river are 30° and 45° respectively. If bridge is at the height of 30m from the bank, then the width of the river is
a) 90m b) 30√3m c) 30(√3+1)m d) 25(√3-1)m
15) The angle of depression of two ships from the top of a lighthouse are 45° and 30° towards east. If the ships are 100m apart, the height of the lighthouse is
a) 20/(√3+1)m b) 20/(√3-1)m c) 50(√3-1)m d) 50(√3+1) m
1b 2c 3b 4c 5a 6a 7d 8a 9b 10b 11c 12b 13d 14c 15c
Short Answer Type Questions
1) A circle artist is climbing a 30m long rope, which is tightly stretched and tide from the top of a vertical pole to the ground. Find the distance of the pole to the peg in the ground, if the angle made by the rope with the ground level is 30°.
2) A tree is a broken by the wind. Find the total height of the tree if the top struck the ground at an angle of 30° and at a distance of 18m from the foot of the pole.
3) A man standing on the top of a vertical tower observes a car moving towards the tower at a uniform speed. if it takes 10 minutes for the angle of depression to change from 30° to 45°, how soon after this will the car reach the tower ?
4) The angles of elevation of the top of a vertical tower from two points, at a distance a and b (a > b) from the base and in the same straight line with it are complementary. Find the height of the tower.
5) If the angle of depression of the top and the bottom of a tower as observed from the top of a h metre high cliff are 30° and 60° respectively, prove that the height of the Tower is 2h/3.
6) The angle of elevation of the top of a vertical Tower PQ from a point X on the ground is 60°. From a point Y, 40m vertically above X, the angle of elevation of the top Q of the tower is 45°. Find the height of the tower PQ and distance PX. (Use √3=1.73)
7) A man observes the angle of elevation of the top of a building to be 30°. He works towards it in horizontal line through its base. On covering 60m, the angle of elevation changey to 60°. Find the height of the building.
1) 15√3 2) 18√3 3) 13.66 min 4) √(ab) 6) 94.8m, 54.8m 7) 52m
Long Answer Type Questions
1) An observer measures angles of elevation of two towers of equal height from a point between the towers. The angle of elevation of the tops of the two towers from this point are 60° and 30°. If this point is at a distance of 120m from first tower , find the distance between the towers.
2) Two towers AB and CD are standing at some distance apart. From the top of tower AB , the angle of depression of the foot of tower CD is 30°. From the top of the tower CD , the angle of depression of the foot of the tower AB is 60°. If the height of the tower CD is 'h' m, then prove that the height of the tower AB is h/3 m.
3) Two poles of equal are standing opposite to each other on either side of the road, which is 80m wide. From a point between them on the road, the angles of the elevation of the top of poles are 60° and 30° respectively. Find the height of poles and the distances of the point from the poles.
4) Ab aeroplane is flying at a height of 300m above the ground. Flying at this height, the angles of depression from the plane to two points on both banks of a river in opposite directions are 45° and 60° respectively. Find the width of the river (Use √3= 1.73)
5) There is a building of height 7m next to a cable tower of unknown height. From the top of the building, the angle of elevation of the top of the tower is 60° and the angle of depression to the foot of the tower is 45°. Find the height of the cable tower.
1) 480m
2) 20√3, 20m
3) 100(3+√3)m
5) 7, (√3+1)m
GRAPHICAL REPRESENTATION OF STATISTICAL DATA
1) Class mark of a class is
a) upper limit + lower limit
b) (upper limit + lower limit)/2
c) (upper limit - lower limit)/2
d) (upper limit + lower limit)
2) For constructing frequency polygon,______ must be calculated.
a) class-marks
b) average of frequency
c) median of frequency
d) cumulative frequency
3) Class mark for the class interval 70-80 is
a) 65 b) 75 c) 85 d) 70
4) Which of the following options represents the frequency polygon for given data ?
Weight (kg) number of student
35.5-40.5 5
40.5-45.5 2
45.5-50.5 12
50.5-55.5 2
55.5-60.5 45
5) Class marks of a particular class is 9.5 and the class size 6, then the class interval is
a) 3.5-15.5 b) 6.5-12.5 c) 12.5-18.5 d) 15.5-27.5
6) ____ of the data is the difference between the maximum and the minimum values of the observation.
a) median b) mean c) range d) mode
7) A dice is thrown 50 times. The table below shows the distribution of outcomes. The frequency of getting a number greater than 2 but less than or equals to 5 is
a) no of dice: 1 2 3 4 5 6
Frequency: 10 12 6 4 8 10
a) 10 b) 22 c) 12 d) 18
8) The given figure shows the height of a group of people. Percentage of people in the height range 149.5-159.5 is equal to:
a) 30% b) 20.5% c) 20% d) 33%
9) The range of the following data is:
26,21,33,17,14,15,27
a) 13 b) 17 c) 15 d) 19
a) Total number of trees is 31.
b) The most common range of height of trees is 70-75 feet.
c) Least common range of height is 85-95 feet
d) The range of height 60-65 feet has minimum number of trees.
1b 2a 3b 4b 5b 6c 7d 8a 9d 10a
Short Answer Type Questions
1) Draw histogram for the following data:
Class-interval frequency
00-10 12
10-20 20
20-30 26
30-40 18
40-50 10
50-60 6
2) A random survey of the number of children of various age groups playing in a park was found as follows:
Age(in yrs) no of children
01-02 5
02-03 3
03-05 6
05-07 12
07-10 9
10-15 10
15-17 4
Draw a histogram to represent the above data.
3) To draw a histogram for the following data, find the adjusted frequency for the class 25-45
Class-interval frequency
05-10 6
10-15 12
15-25 10
25-45 8
45-75 15
4) Draw histogram for the following data:
Marks no of students
00-20 10
20-40 15
40-60 20
60-100 25
5) Construct the more cumulative frequency table for the following data:
Class-interval frequency
00-10 3
10-20 8
20-30 12
30-40 14
40-50 10
50-60 6
60-70 5
70-80 2
6) Make a less than cumulative frequency table for the following data:
Class-interval frequency
60-70 2
70-80 5
80-90 12
90-100 31
100-110 39
110-120 10
120-130 4
7) During the medical check up of 35 students of a class, their heights were recorded as follows:
Height in 'cm' no of students
Less than 38 0
Less than 40 3
Less than 42 5
Less than 44 9
Less than 46 14
Less than 48 28
Less than 50 32
Less than 52 35
Draw the ogive for the above data.
Long Answer Type Questions
1) The given histogram depicts the daily wages of workers in a factory. Construct the frequency table.
2) Following is the data of maximum temperatures recorded in 20 days in a city: 10°C, 12°C, 15°C, 40°C, 25°C, 20°C, 30°C, 28°C, 25°C, 27°C, 18°C, 32°C, 34°C, 27°C, 29°C, 16°C, 36°C, 35°C, 33°C, 22°C.
Prepare the Frequency table and draw a histogram to represent the above data.
Read the histogram and answer the questions that follow:
a) How many teachers are in the age group of 45-50 years?
b) How many teachers are above 45 years of age?
c) How many teachers are below 35 years of age ?
d) Which age group has the maximum number of teachers?
e) Which age group has the minimum number of teachers?
4) The following distribution table shows the IQ level of 270 candidates appearing for a competitive exam.
IQ. No of candidates
55-69 20
69-83 50
83-97 75
97-111 75
111-125 50
Draw a histogram for this distribution.
5) Draw an ogive for the following data:
Marks no of students
00-10 7
10-20 10
20-30 23
30-40 51
40-50 6
50-60 3
3 8 22 30 30-35 45-50
MEASURE OF CENTRAL (MEAN)
1) If the classes of a frequency distribution are 0-10, 10-20, 20-30,....50-60, then the size of each class is
a) 9 b) 10 c) 11 d) 5.5
2) The measure of central tendency of a statistical data which takes into account all the data is :
a) mean b) median c) mode d) range
3) For a group frequency distribution, we use bar X = ∑fx/∑f, here x stands for :
a) width of a class
b) lower limit of a class
c) class mark of a class d) none
4) For a grouped frequency distribution, we use bar X = A+ ∑fd/∑f to find the mean using shortcut method: here d stands for
a) width a class
b) midpoint of a class
c) frequency of a class
d) deviation of class mark from assumed mean .
5) For a grouped frequency distribution, we use bar X = A + ∑ft/∑f Here ∑f stands for:
a) sum of frequencies
b) Sum of class-marks
c) sum of upper limits of the classes.
d) sum of lower limits of the classes.
6) For a grouped frequency distribution we used bar X = A + ∑ft/∑f to find the mean using step deviation method. Here t is given by:
a) x - A B) (x+ A)/h c) (x - A)/h d) xA/h
7) While computing mean of a grouped data we assume that the frequency are:
a) evenly distributed over the classes
b) centred at the class marks of the classes
c) centred at the upper limits of the classes.
d) Centred at the lower limits of the classes.
8) If xᵢ's are the midpoints of the class intervals of grouped fᵢ's are corresponding frequencies and bar X is the mean, then ∑(fᵢxᵢ - bar X) is equal to:
a) 0 b) 1 c) -1 d) 2
9) The mean of the following frequency distribution to the nearest integer is
Class: 0-2 2-4 4-6 6-8
F: 2 1 4 3
a) 4 b) 5 c) 6 d) 7
10) The mean of the following distribution is
Clar: 1-3 4-6 7-9 10-12
F: 5 2 1 4
a) 10 b) 8 c) 6 d) 5
1b 2a 3c 4d 5a 6c 7b 8a 9b 10c
Short Answer Type Questions
1) Find the mean of the following data:
C-I: 0-10 10-20 20-30 30-40 40-50
F: 12 16 6 7 9 22
2) Find the mean of the following data using direct method:
C-I: 0-4 5-9 10-14 15-19
F: 2 8 7 3 9.75
3) Find the mean of the following data using short cut method:
C-I: 0-20 20-40 40-60 60-80
F: 10 8 15 7 39.5
4) Find the mean of the following data using step deviation method:
C-I: 1-3 3-5 5-7 7-9 8-11
F: 7 8 2 2 1 4.2
5) Find the mean of the following distribution:
Marks no of students
Less than 20 4
Less than 40 16
Less than 60 24
Less than 80 30 40.66
6) Find the mean of the following data:
Height (in cm) no of plants
More than 10 50
More than 20 36
More than 30 18
More than 40 5 26.8
7) If the mean of the following distribution is 22, then find the value of f:
Class 0-10 10-20 20-30 30-40 40-50
F: 12 16 6 f 9 7
8) The daily incomes of a group of 10 labourers are tabulated as below. Find the mean daily income of the labourers.
Income(in Rs) no of labourers
100-200 2
200-300 4
300-400 3
400-500 1 Rs280
Long Answer Type Questions
1) The mean of the following frequency distribution is 62.8 and the sum of all frequencies is 50. Compute the missing frequencies a and b.
Class Frequency
00-20 5
20-40 a
40-60 10
60-80 b
80-100 7
100-120 8
Total 50 8,12
2) The daily expenditure of 100 families is given below. Calculate x and y, if the mean daily expenditure is Rs188.
Expenditure(in Rs) no of families
140-160 5
160-180 25
180-200 x
200-220 y
220-240 5 50,15
3) Using the assumed mean method, find the mean of the following data:
Class: 0-10 10-20 20-30 30-40 40-50
F: 7 8 12 13 10 27.2
4) The following table gives the wages (in Rs) of workers (per day) in a factory:
Wages No of workers
130-134 5
134-138 8
138-142 14
142-146 12
146-150 11
Calculate the mean wage of the workers of the factory by using assumed -mean method (short cut) method. Rs 141.28
5) Calculate the mean of the following frequency distribution, using the step deviation method.
Class frequency
00-50 17
50-100 35
100-150 43
150-200 40
200-250 21
250-300 24 148.61
6) By using step deviation method, compute the arithmatic mean for the following data:
a) Marks. no of students
Less than 10 14
Less than 20 22
Less than 30 37
Less than 40 58
Less than 50 67
Less than 60 75 28.6
7) By using step deviation method, find the mean marks obtained by a student from the following data:
Marks. No of students
0 and above 80
10 and above 77
20 and above 72
30 and above. 65
40 and above 55
50 and above 43
60 and above 28
70 and above 16
80 and above 10
90 and above 8
100 and above 0 51.75
Median, Quartiles and Mode
1) From histogram, we can estimate.
a) mean of the data
b) median of the data
c) mode of the data
d) upper quartile of the data
a) 72 b) 21 c) 48 d) 36
3) Which of the following is correct?
a) mode= 3 median - 2 mean
b) mode= 2 median - 3 mean
c) mode= 3 median + 2 mean
d) mode= 2 mean - 3 median
a) 10 b) 15 c) 20 d) 25
5) The class mark of the median class of the given distribution is
Class Cumulative frequency
00-10 8
10-20 10
20-30 17
30-40 26
40-50 30
a) 15 b) 25 c) 30 d) 35
6) Semi-inter quartile range is given by
a) (Q₃ + Q₁)/2
b) (Q₃ - Q₁)/2
c) (Q₃ + Q₁)
d) (Q₃ + Q₁)/3
7) The median of the data 8,5,-2,4,0,7,10,6,3 is
a) 0 b) 5 c) -2 d) 10
8) The mode of the given observation 10,22,15,18,17,15,10,21,9,6,10,16,15,4,15 is
a) 15 b) 10 c) 22 d) 6
9) From the data 37,35,30,34, 40,39,38,36 if 35 is removed, the median increases by:
a) 0.5 b) 1 c) 2 d) 35
10) The median of the data 78,56,22,34,45,54,39,68,54,84 is
a) 53 b) 54 c) 55 d) 56
11) In a grouped frequency distribution, the class with maximum frequency is called
a) mean class b) media class c) modal class d) quartile class
12) Quartile divide the whole set of observation into ____ equal parts.
a) two b) three c) four d) ten
13) The middle Quartile is also known as
a) median b) mode c) mean d) class mark
14) The difference between upper quartile (Q₃) and lower quartile ( Q₁) is called
a) range
b) semi inter quartile range
c) interquartile range d) limits
15) If the median of the data 24,25,26, x+2, x +3, 30,31,34 arranged in order is 27.5, then the value of x is
a) 25 b) 27 c) 28 d) 30
1c 2a 3a 4b 5d 6b 7b 8a 9a 10b 11c 12c 13a 14c 15a
Short Answer Type Questions
1) The median of the following obser11,12,14,18, (x +4), 30, 32,35,41 arranged in ascending order is 24. Find x.
2) Find the median of 17,26,60,45,33,32,29,34,56. If 26 is replaced by 62, find the new median.
3) The marks scored by 16 students in a class test are: 3,6,8,13,15,5 , 21,23,17,10, 9, 1,20,21, 18,12. Find
a) the median
b) lower quartile
c) upper quartile
4) The mean of the observations 23, (x -5),36, (x -9), (x -1) and 28 is 21. Find the value of x and hence find the median.
5) The median of the observation 11,12,14, (x -2), (x +4), (x +9),32,38 and 47 arranged in ascending order is 24. Find the value of x and hence find the mean,
1) 20 2) 33;34 3) 12.5,6,18 4) 18,20 5) 20,25
Long Answer Type Questions
1) Find the median from the following frequency distribution:
Wages. No of workers
280 12
320 16
360 20
440 17
480 13
550 11
2) Find the median from the following frequency distribution:
Weight no of students
56 6
59 8
62 13
64 12
66 9
70 6
3) Calculate first quartile, third quartile and quartile range from the following data:
Weight: 4 5 6 8 11 13 14
F: 2 4 5 7 3 2 1
4) In a class of 40 students, marks obtained by the students in a class test(out of 10) are given below:
Marks no of students
1 1
2 2
3 3
4 3
5 6
6 10
7 5
8 4
9 3
10 3
Calculate the following for the given distribution:
a) median
b) mode
5) The distribution given below shows the marks obtained by 25 students in an aptitude test. Find the median and mode of the distribution.
Marks: 5 6 7 8 9 10
F: 3 9 6 4 2 1
6) A mathematical aptitude test of 50 students was recorded as follows:
Marks no of students
50-60 4
60-70 8
70-80 14
80-90 19
90-100 5
Draw a histogram for the above data using a graph paper and locate the mode.
7) Draw a histogram for the following frequency distribution and find mode from the graph.
Class frequency
00-05 2
05-10 5
10-15 18
15-20 14
20-25 8
25-30 5
8) (Use a graph paper for this question.) The daily pocket expenses of 200 students in a school are given below:
Expenses. Frequency
00-05 10
05-10 14
10-15 28
15-20 42
20-25 50
25-30 30
30-35 14
35-40 12
Draw a histogram representing the above distribution and estimate the mode from the graph.
1) Rs360 2) 63kg 3) 5,8,3 lbs
4) 6,6
5) 7,6
6) 82.5 7) 14 8) 21
Long Answer Type Questions - II
1) The table shows the distribution of the scores obtained by 160 shooters in a shooting competition. Use a graph sheet and draw an ogive for the distribution. (take 2cm= 10 score on the x-axis and 2cm = 20 shooters on the y-axis).
Scores no of shooters
00-10 9
10-20 13
20-30 20
30-40 26
40-50 30
50-60 22
60-70 15
70-80 10
80-90 8
90-100 7
Use your graph to estimate the following:
a) the medium
b) the interquartile range
c) The number of shooters who obtained a score of more than 85%.
2) The daily wages of 80 workers in a project are given below:
Wages No of workers
400-450 2
450-500 6
500-550 12
550-600 18
600-650 24
650-700 13
700-750 5
Use a graph paper to draw an ogive for the above distribution. (Use a scale of 2cm = Rs50 on x-axis and 2cm = 10 workers on y-axis). Use your ogive to estimate:
a) the median wage of the workers.
b) the lower quartile wage of workers.
c) the number of workers who earn more than Rs625 daily.
3) Use graph paper for this question.
A survey regarding height (in cm) of 60 boys belonging to class 10 of a school was conducted. The following data was recorded:
Height no of boys
135-140 4
140-145 8
145-150 20
150-155 14
155-160 7
160-165 6
165-170 1
Taking 2cm = height of 10cm along one axis and 2cm= 10 boys along the other axis, draw an ogive for the above distribution. Use the graph to estimate the following:
a) the median
b) lower quartile
c) if above 158cm is considered as the tall boys of the class, find the number of boys in the class who are tall.
4) 40 students enter for a game of shot-put competition. The distance thrown (in metres) is recorded below:
Distance no of students
12-13 3
13-14 9
14-15 12
15-16 9
16-17 4
17-18 2
18-19 1
Use a graph paper to draw an ogive for the above distribution.
Use a scale of 2cm= 1m on one axis and 2cm = 5 students on the other axis.
Hence using your graph find
a) the median
b) upper quartile
c) the number of students who cover a distance which is above 33/2 m.
1) 44 31 11
2) Rs600, Rs 550, 29
3) 149cm, 146, 9
4) 14.6m, 15.6m, 5
PROBABILITY
Multiple Choice Questions
1) If an event cannot occur, then its Probability is:
a) 1 b) 3/4 c) 1/2 d) 0
2) Which of the following cannot be the probability of an event?
a) 1/3 b) 0.1 c) 3% d) 17/16
3) An event is very unlikely to happen. Its Probability is closest to:
a) 0.0001 b) 0.001 c) 0.01 d) 0.1
4) If the probability of an event is p, The probability of its complementary event will be:
a) p -1 b) p c) 1- p d) 1- 1/p
5) Which of the following can be the probability of an event?
a) -0.04 b) 1.04 c) 18/23 d) 8/7
6) If P(A) denotes the probability of an event A, then
a) P(A) <0 b) P(A)>1 c) 0≤ P(A)≤ 1 d) -1≤ P(A)≤ 1
7) A card is selected from a deck of 52 cards. The probability of its being a red face card is :
a) 3/26 b) 3/13 c) 2/13 d) 1/2
8) The Probability that a non leap year selected at random will contains 53 Sundays is:
a) 1/7 b) 2/7 c) 3/7 d) 5/7
9) When a die is thrown, the probability of getting an odd number less than 3 is:
a) 1/6 b) 1/3 c) 1/2 d) 0
10) If a letter is drawn at random from the letters inword ERROR, then the letters which have equal probability of being drawn are:
a) E and O b) R and E c) O and R d) E, R and O
11) From the data (1, 4, 9, 16, 25, 29) if 29 is removed , then the probability of a getting a number which is neither a prime nor a composite is:
a) 2/5 b) 1/5 c) 3/5 d) 4/5
12) A game of chance consisting of spinning an arrow which comes to rest pointing at one of the numbers 1, 2, 3, 4, 5, 6, 7, 8 and these are equally likely outcomes. Then, the probability that it will point at a prime number is
a) 1/8 b) 5/8 c) 3/8 d) 1/2
13) It is given that in a group of three students, the probability of two students not having the same birthday is 0.991. then, the probability of the two students having the same birthday is :
a) 0.009 b) 0.001 c) 0.990 d) 0.007
14) If the probability of success is 38%, then the probability of failure is:
a) 38% b) 62% c) 52% d) 68%
15) In a flower bed, every third plant is a rose plant. if a child picks a flower, then the probability of the flower being other than rose is :
a) 1/5 b) 1/3 c) 2/3 d) 2/5
16) Two dice are thrown together. The probability of getting the same number on both the dice is
a) 1/2 b) 1/3 c) 1/6 d) 1/12
17) A card is drawn from a pack of 52 cards. The event E is that the card is not an ace of hearts. The number of outcomes favourable to E is:
a) 4 b) 14 c) 21 d) 51
18) The probability of a getting a bad egg in a lot of 400 is 0.035. The number of bad eggs in the lot is :
a) 7 b) 14 c) 21 d) 28
19) A girl calculates that the probability of her winning the first prize in a lottery is 0.08. if 6000 tickets are sold, how many tickets has she bought ?
a) 40 b) 240 c) 480 d) 750
20) One ticket is drawn at random from a bag containing tickets numbered 1 to 40. The probability that the selected ticket has a number which is a multiple of 5 is :
a) 1/5 b) 3/5 c) 4/5 d) 1/3
21) Someone is asked to take a number from 1 to 100. The probability that it is prime is:
a) 1/5 b) 6/25 c) 1/4 d) 13/50
22) In a single throw of 2 dies, the probability of getting 6 as a product is:
a) 4/9 b) 2/9 c) 1/9 d) 5/9
23) The probability of getting an even number, when a die is thrown once, is
a) 1/2 b) 1/3 c) 1/6 d) 5/6
24) Many birds were sitting on a tree. Every seventh bird was a sparrow. A bird flew away. What is the probability that the bird was not a sparrow?
a) 5/7 b) 3/7 c) 6/7 d) 1/7
25) A box contains cards numbered 6 to 50. A card is drawn at random from the box. The probability that the drawn card has a number which is a perfect square is
a) 1/45 b) 2/15 c) 1/9 d) 4/45
1d 2d 3a 4c 5c 6a 7a 8a 9a 10a 11b 12d 13a 14b 15c 16c 17d 18b 19c 20a 21c 22c 23a 24c 25c
Short Answer Type Questions
1) A Jar contains 24 marbles, some are green and other are blue. If a marble is drawn at a random from the jar, the probability that it is given is 2/3. Find the number of blue marbles in the jar. 8
2) A bag contains 100 identical marble stones numbered from 1 to 100. What is the probability of drawing a marble having a number divisible by both 4 and 5. 1/20
3) A card is drawn at random from a well shuffled pack of 52 cards. Find the probability that the card is black or king. 7/13
4) From a set of 17 cards numbered 1,2,3,....17, one card is drawn at random. What is the probability that number on the drawn card is multiple of 3 or 7? 7/17
5) There are 5 green, 6 black and 7 white balls in a bag. A ball is drawn at random from the bag. Find the probability that it is not a white. 11/18
6) If an unbiased dice is thrown 5 times and every throw resulted in a 6, what is the probability of getting a 6 on the sixth throw. 1
7) A card is drawn at random from a well shuffled deck of 52 cards. What is the probability that the card drawn is a diamond? 1/4
8) A ball is chosen from a bag that contains 30 balls numbered from 1 to 30. What is the probability that the ball chosen will have a number that is divisible by 3? 1/3
Long Answer Type Questions
1) 5 defective glasses are accidentally mixed with 20 good ones. The good glasses and the defective one look same. If a glass is chosen at random, what is the probability that the chosen glass is good? 4/5
2) A box of 15 chocolates has 6 that contains caramel. 5 that contain nuts and 4 that contain cashews. If you randomly selected a chocolate, what is the probability that you will get one that contains nuts? 1/3
3) A bag contains red, blue and green balls. It is twice as likely to pick a blue ball as a compared to a red ball, it is thrice as likely to pick a green ball as compared to a red ball. What is the probability of picking a green ball? 1/2
4) A dice is thrown twice. Find the probability of getting a bigger value on the first throw. 5/12
5) A box contains 3 black balls, 4 red balls and 3 green balls. All the balls are identical in shape and size. Rohit takes out a ball from the bag without looking into it. What is the probability that the ball drawn is a black or green ball. 3/5
6) Two dice are thrown at the same time. Write down all the outcomes. Find the probability that the sum of the two number appearing on the dice is.
a) 8 b) 13 c) lesser than or equal to 12. 5/36, 0, 1
7) A card is drawn at random from a pack of cards. Find the probability of getting
a) a red king. 1/26
b) a queen or a Jack. 2/13







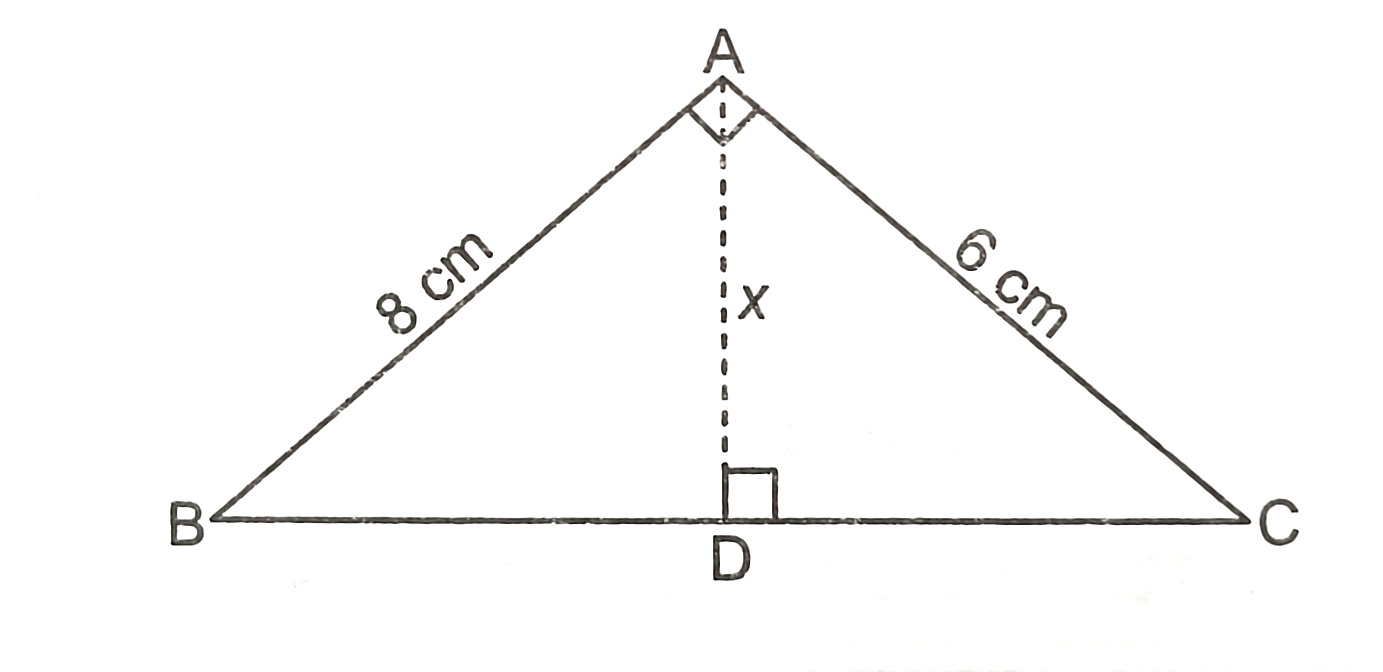

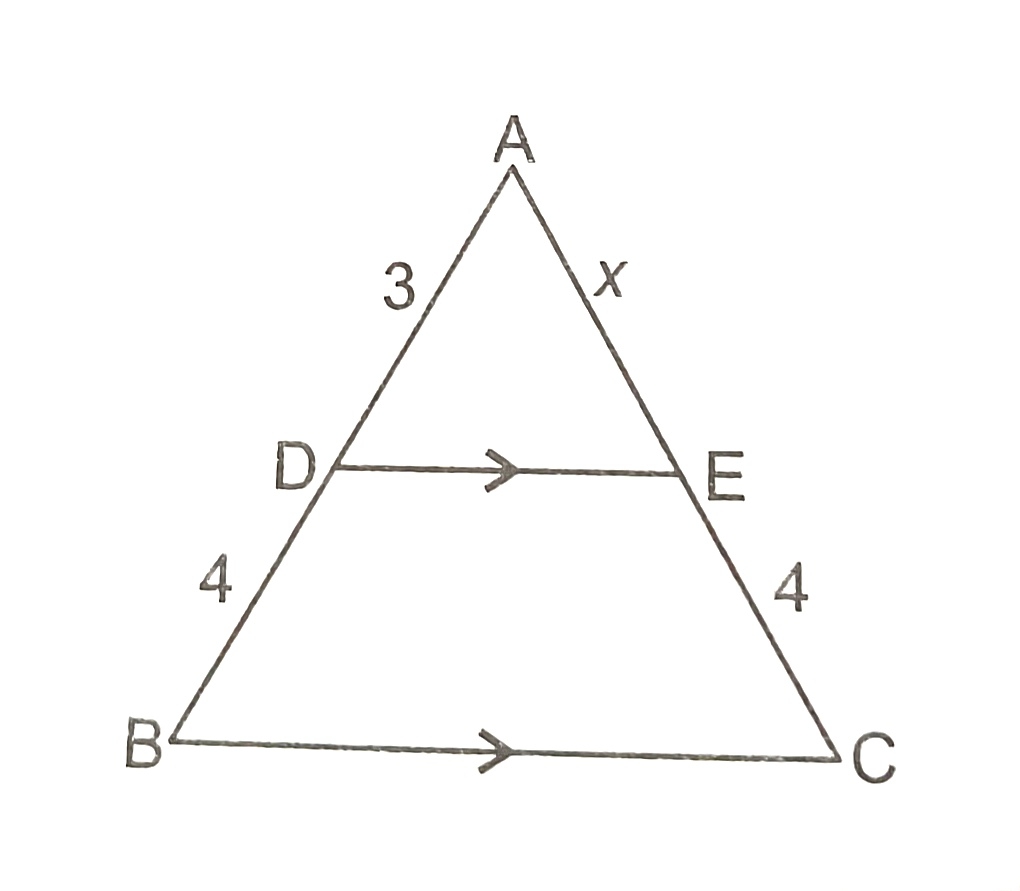






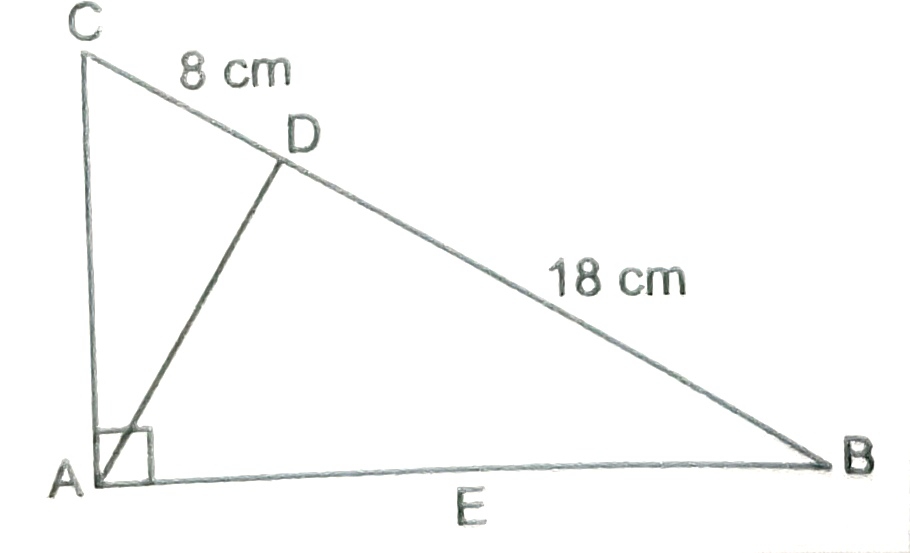






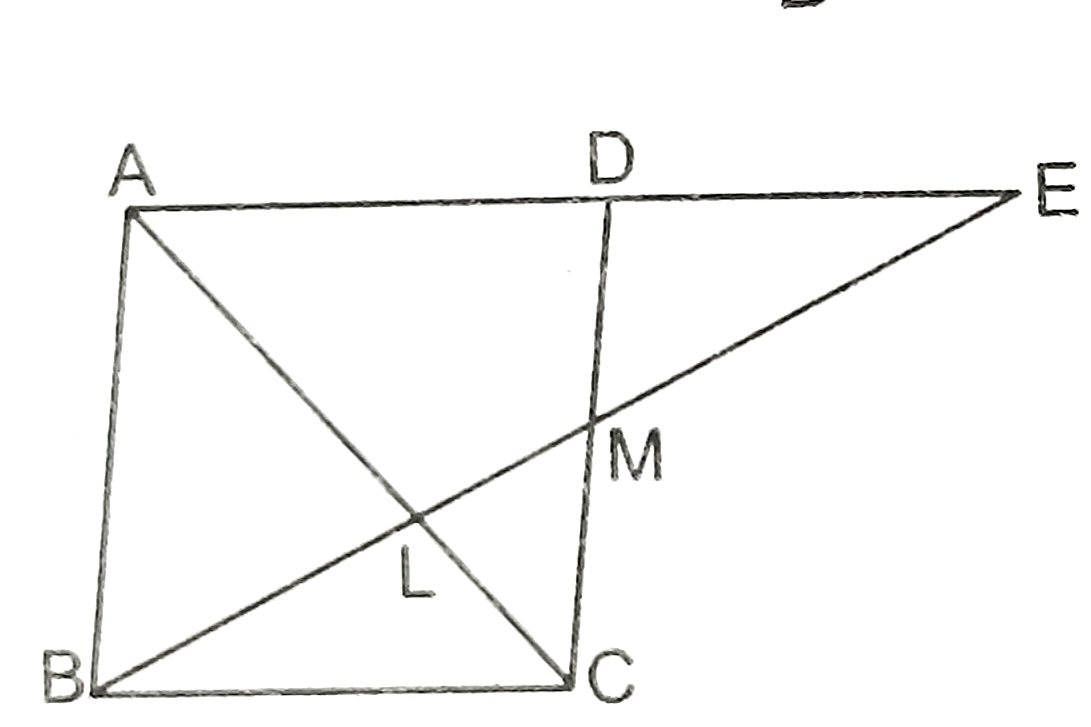



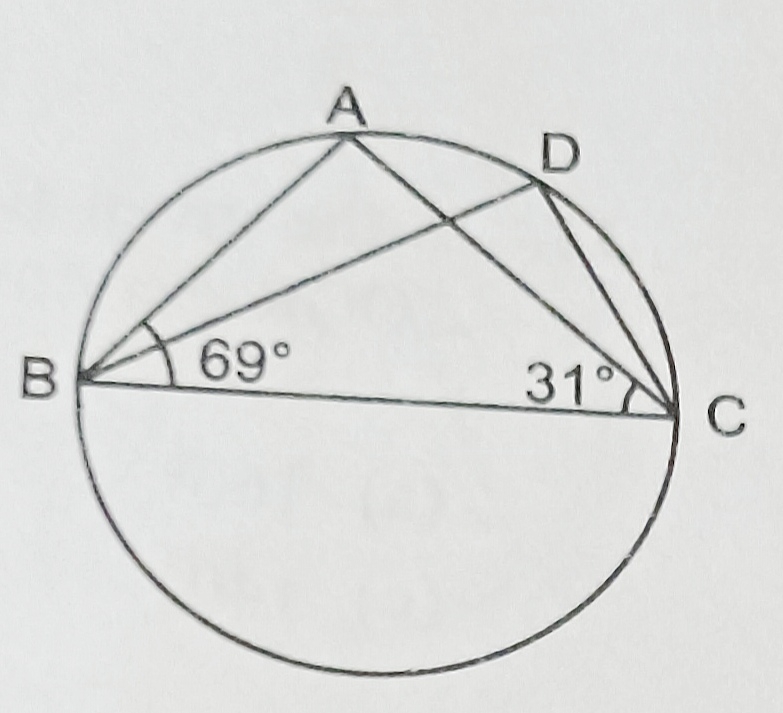
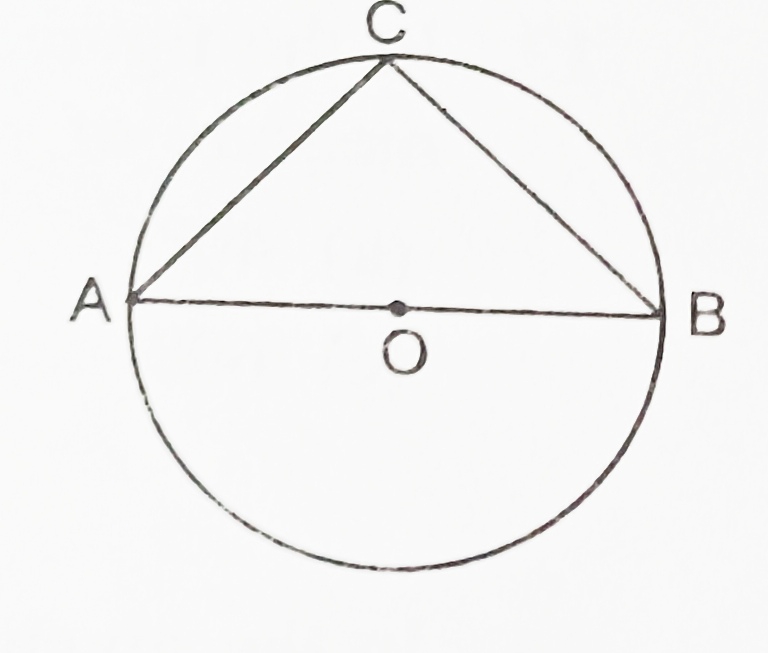
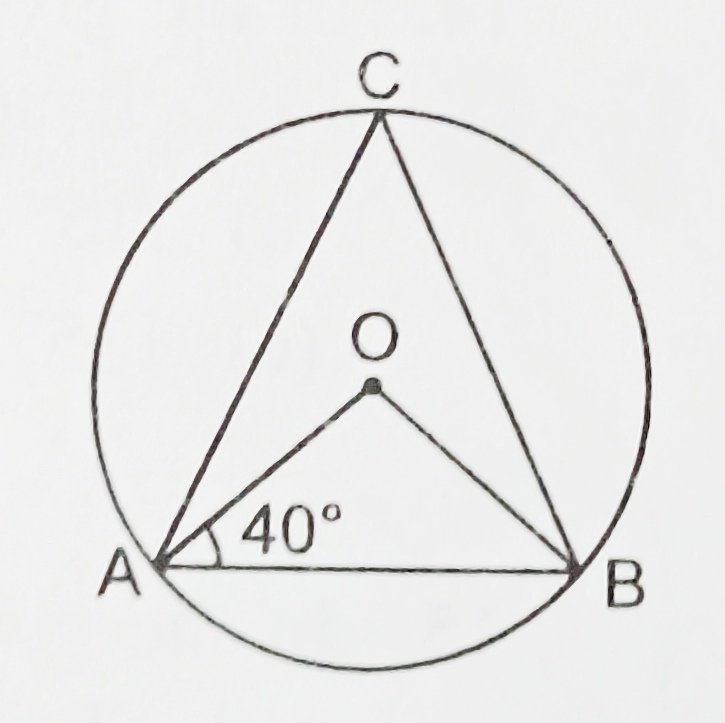



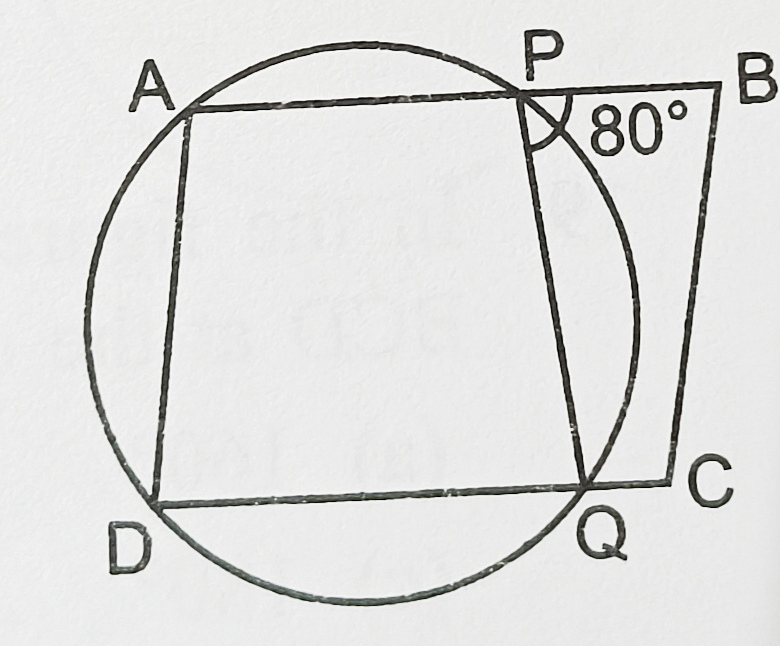
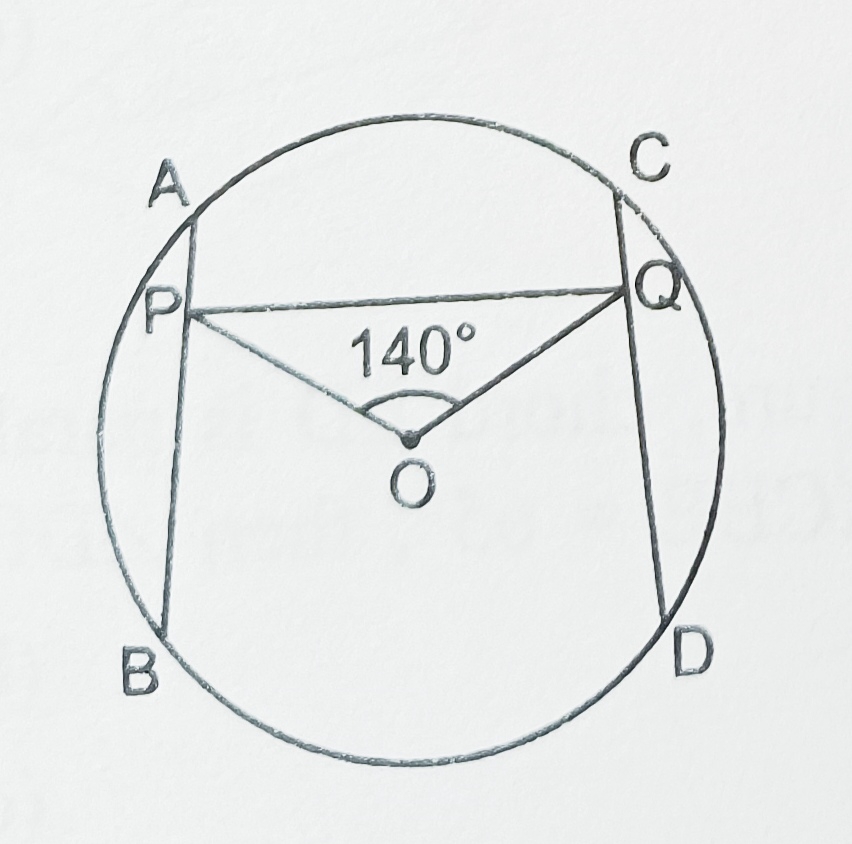














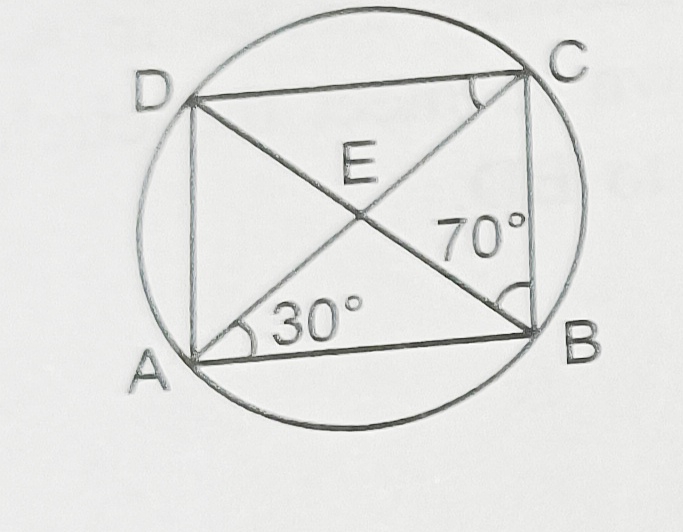
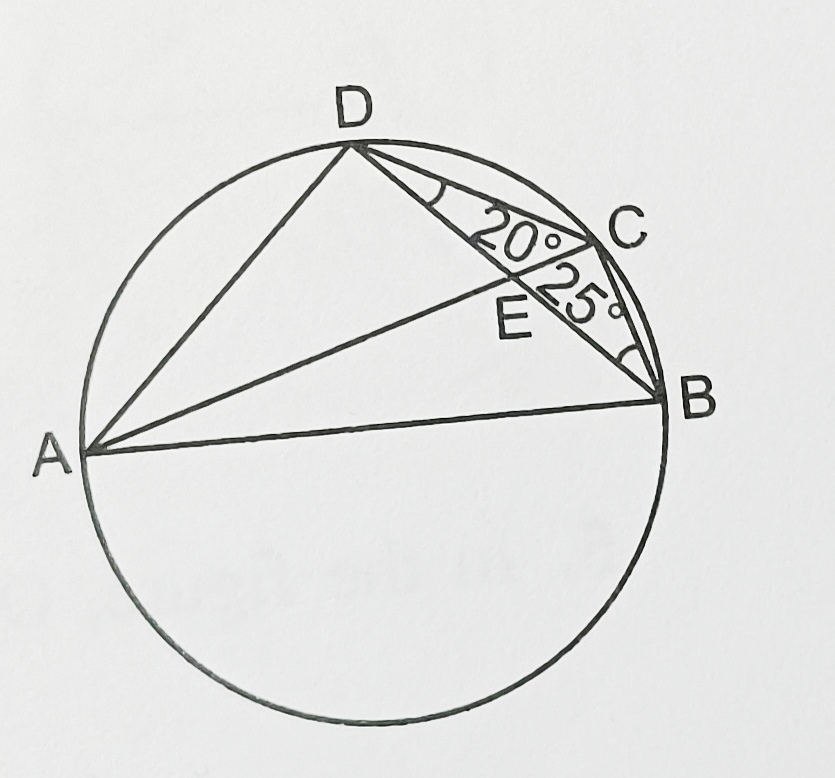





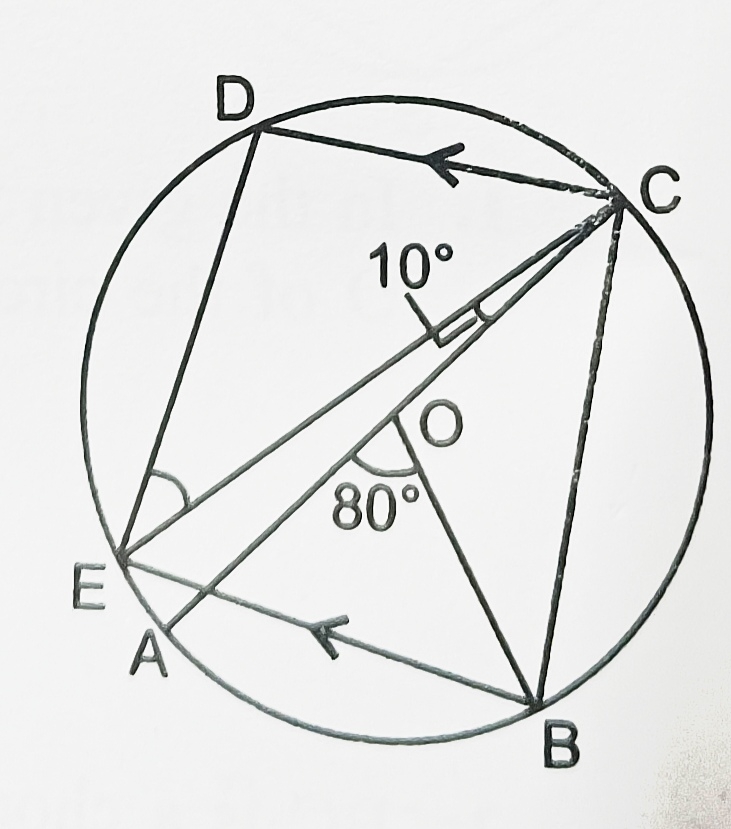







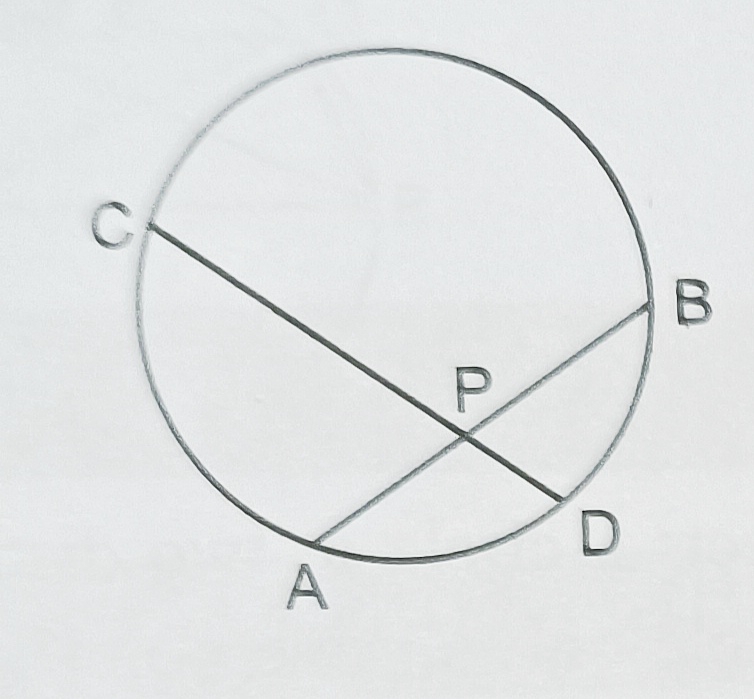





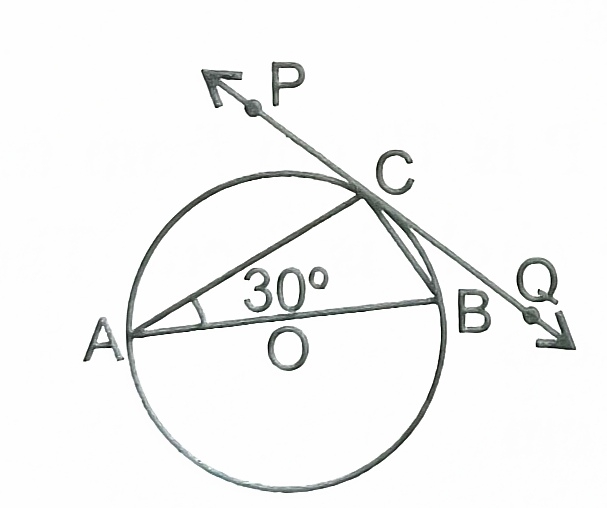





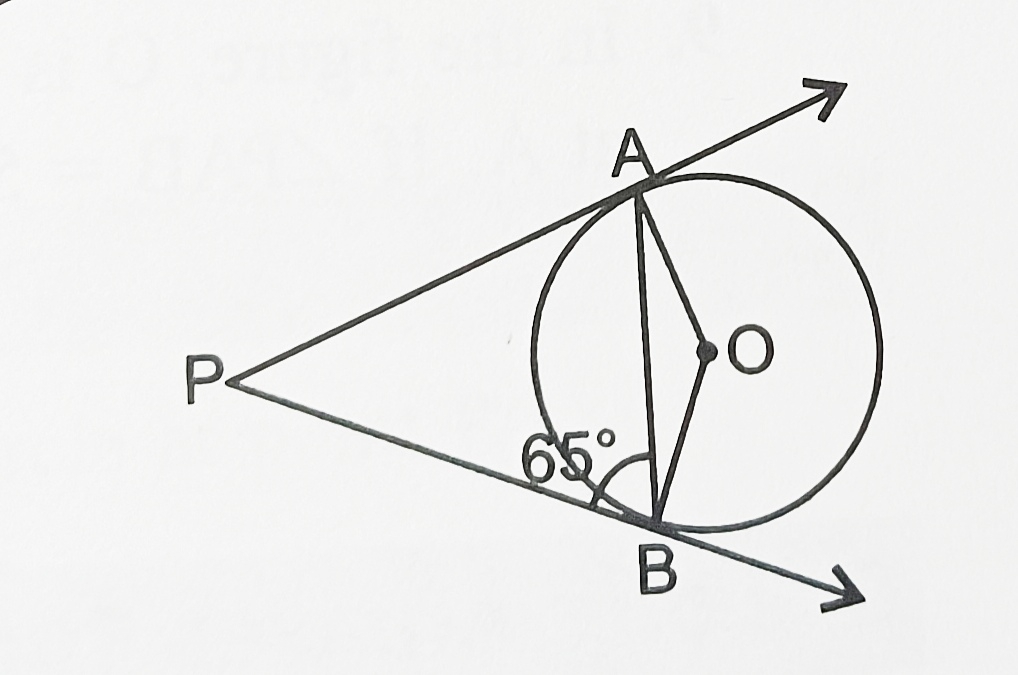

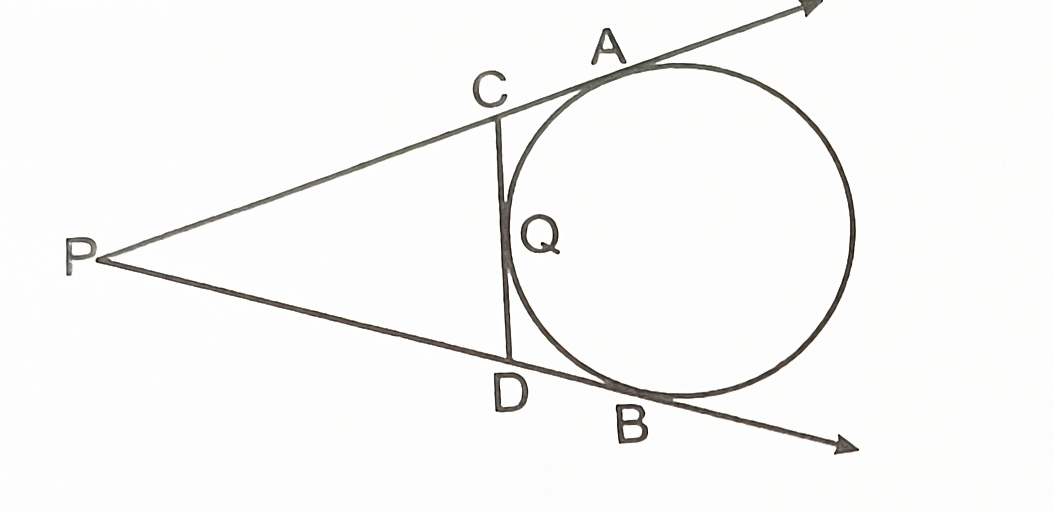







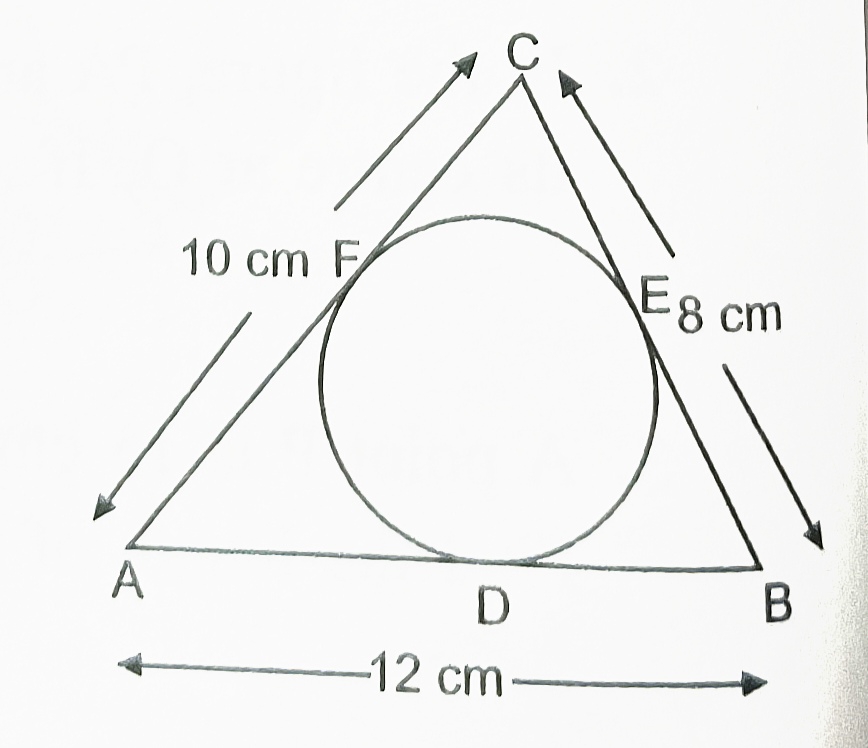






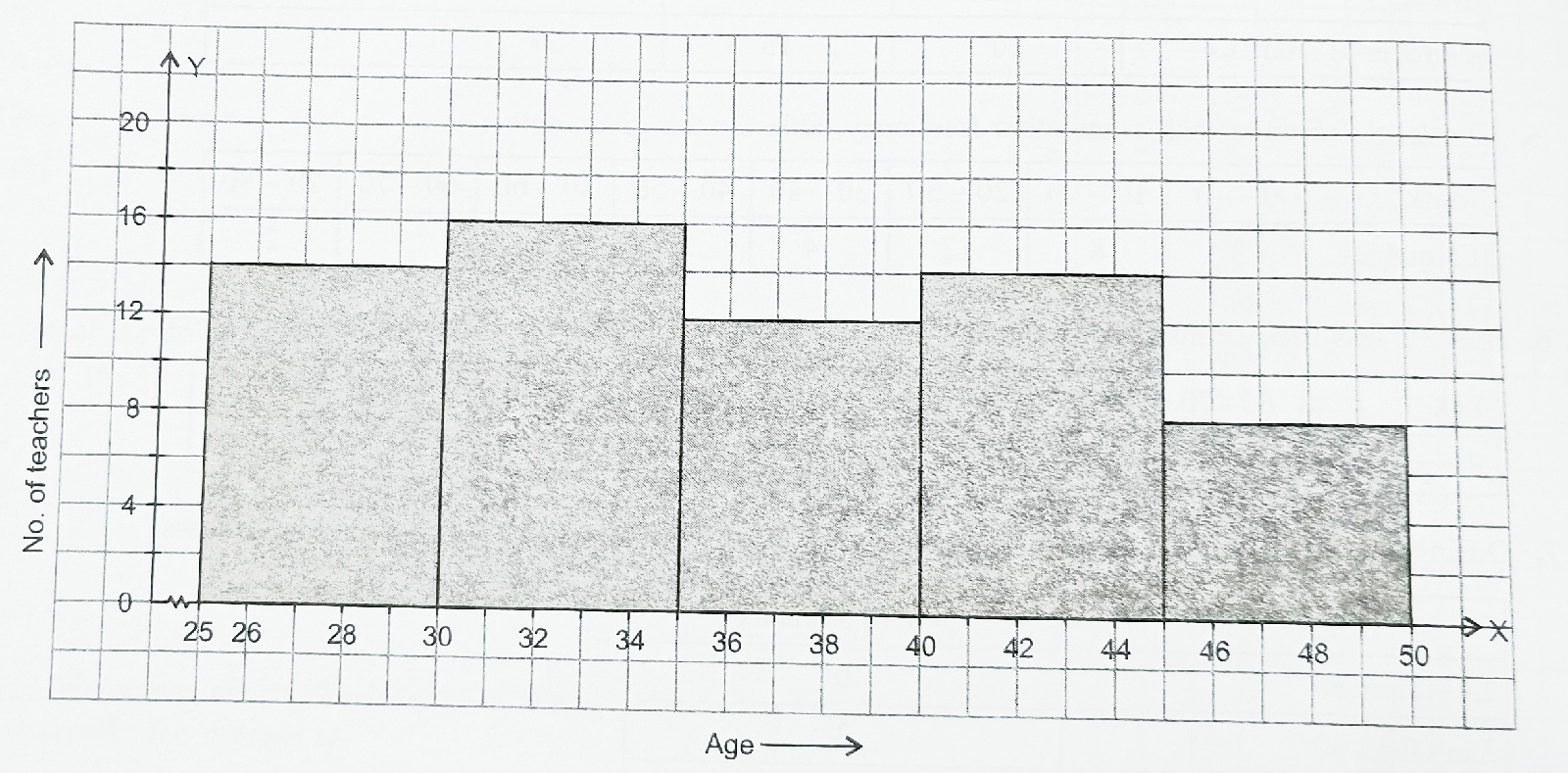
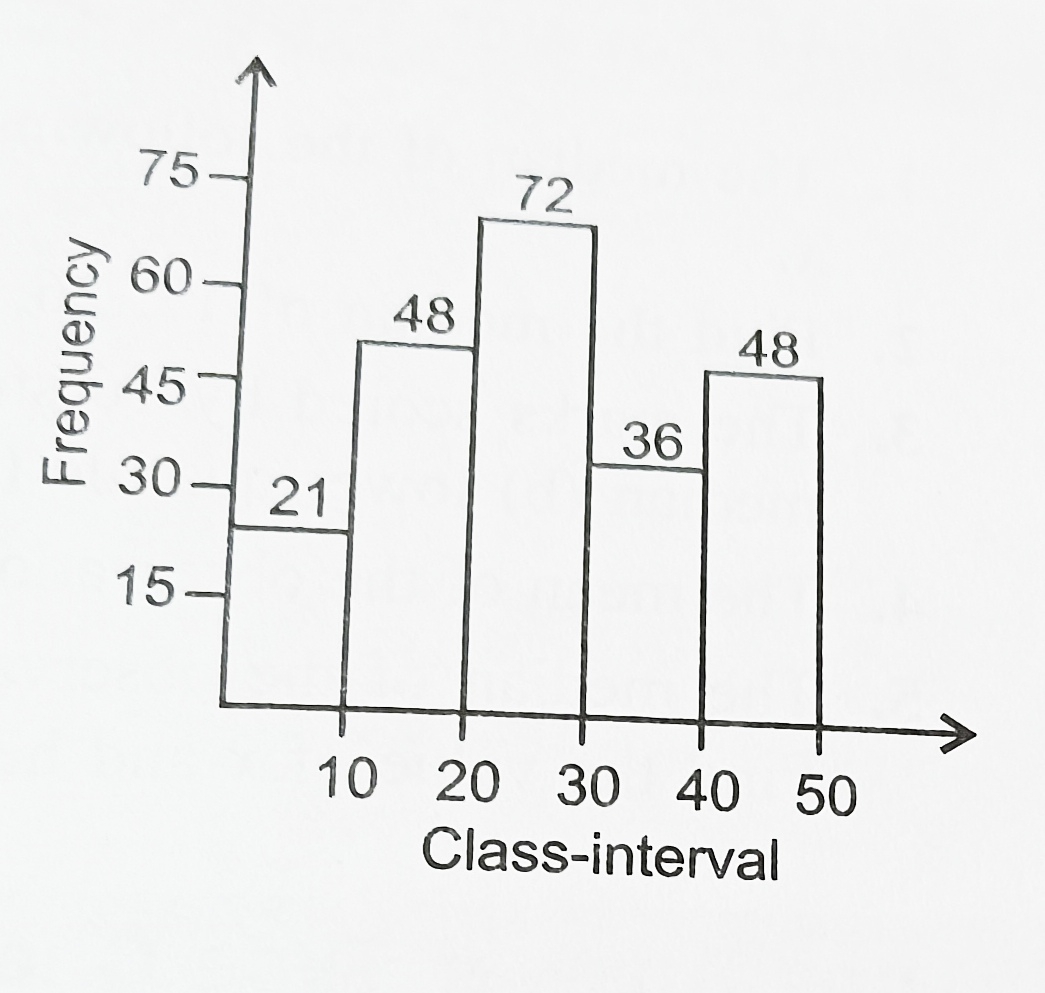
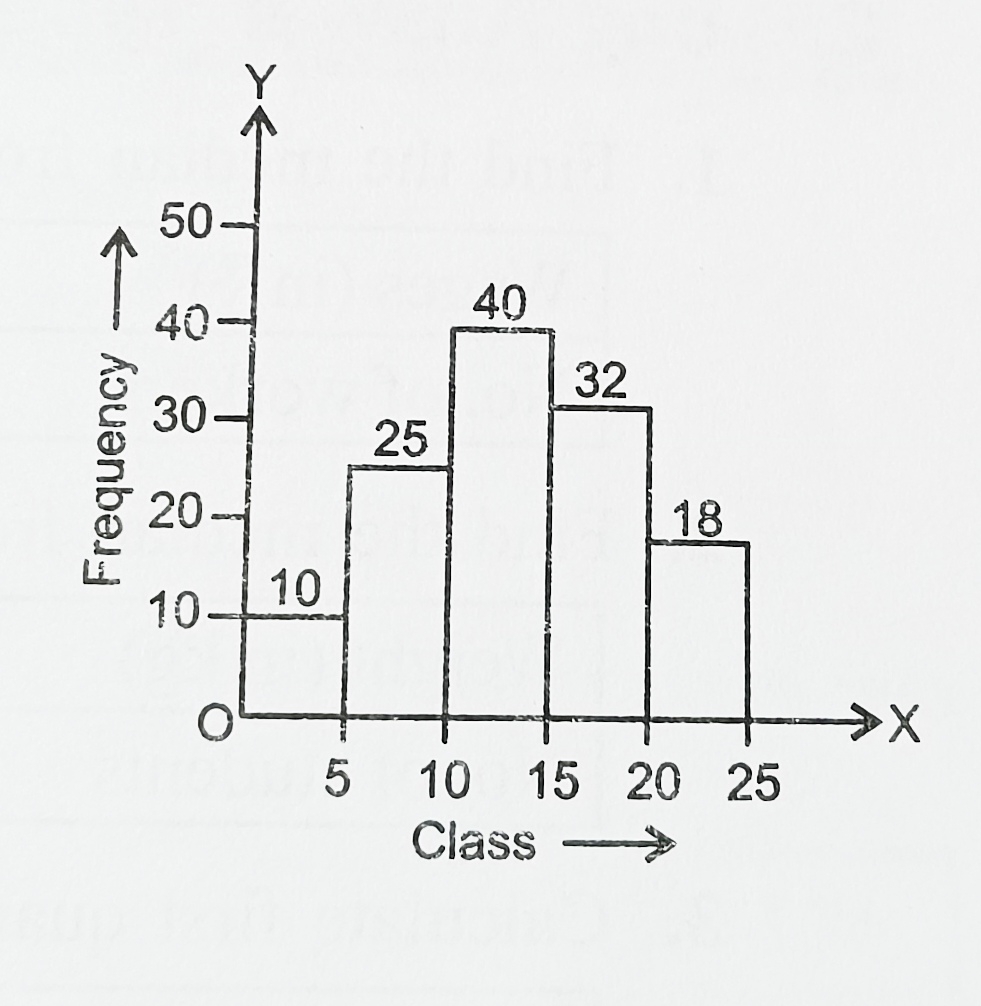
Comments
Post a Comment